Algebra Worksheets
Click any of the links below to download the corresponding worksheet and answer key.
How to Download/Print | Jump to a Topic:
▶️ Commutative property of Multiplication (A)
▶️ Commutative property of Multiplication (B)
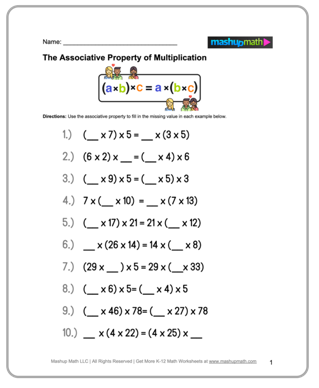
▶️ Associative property of Multiplication (A)
▶️ Associative property of Multiplication (B)
▶️ Practice with the Distributive Property (Algebra) (A)
▶️ Practice with the Distributive Property (Algebra) (B)
▶️ Practice with the Distributive Property (Algebra) (C)
▶️ Practice with Absolute Values (A)
▶️ Practice with Absolute Values (B)
▶️ Practice with Absolute Values (C)
▶️ Practice: Order of Operations with Exponents (A)
▶️ Practice: Order of Operations with Exponents (B)
▶️ Practice: Order of Operations with Exponents (C)
▶️ Practice: Order of Operations with Nested Parenthesis (A)
▶️ Practice: Order of Operations with Nested Parenthesis (B)
▶️ Practice: Order of Operations with Nested Parenthesis (C)
▶️ Extra practice (order of operations up to 6 terms) (A)
▶️ Extra practice (order of operations up to 6 terms) (B)
▶️ Extra practice (order of operations up to 6 terms) (C)
▶️ Extended Practice: Order of Operations
▶️ Finding percent increase/decrease (A)
▶️ Finding percent increase/decrease (B)
▶️ Solving simple proportions (A)
▶️ Solving simple proportions (B)
▶️ Solving simple proportions (C)
▶️ Factoring numbers (up to 100) (A)
▶️ Factoring numbers (up to 100) (B)
▶️ Factoring numbers (up to 100) (C)
▶️ Finding Greatest Common Factor (GCF) (A)
▶️ Finding Greatest Common Factor (GCF) (B)
▶️ Finding Least Common Multiple (LCM) (A)
▶️ Finding Least Common Multiple (LCM) (B)
▶️ Extended Practice: Finding GCF and LCM
▶️ Plotting Points (All Quadrants) (A)
▶️ Plotting Points (All Quadrants) (B)
▶️ Reading Points (All Quadrants) (A)
▶️ Reading Points (All Quadrants) (B)
▶️ Translations on the coordinate plane (A)
▶️ Translations on the coordinate plane (B)
▶️ Rotations on the coordinate plane (A)
▶️ Rotations on the coordinate plane (B)
▶️ Reflections on the coordinate plane (A)
▶️ Reflections on the coordinate plane (B)
▶️ Negative or zero exponents (A)
▶️ Negative or zero exponents (B)
▶️ Multiplying Exponents with the Same Base (A)
▶️ Multiplying Exponents with the Same Base (B)
▶️ Dividing Exponents with the Same Base (A)
▶️ Dividing Exponents with the Same Base (B)
▶️ Practice with Scientific Notation (A)
▶️ Practice with Scientific Notation (B)
▶️ Practice with Scientific Notation (C)
▶️ Extended Practice: Scientific Notation (A)
▶️ Practice with square roots (A)
▶️ Practice with square roots (B)
▶️ Practice with square roots (C)
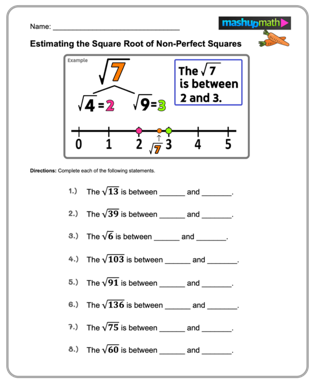
▶️ Estimating Square Roots of Non-Perfect Squares (A)
▶️ Estimating Square Roots of Non-Perfect Squares (B)
▶️ Adding and Subtracting Radicals (A)
▶️ Adding and Subtracting Radicals (B)
▶️ Multiplying and Dividing Radicals (A)
▶️ Multiplying and Dividing Radicals (B)
▶️ Practice with the Pythagorean Theorem (A)
▶️ Practice with the Pythagorean Theorem (B)
▶️ Practice with the Pythagorean Theorem (C)
▶️ Practice with the Pythagorean Theorem (D)
▶️ Practice with the Pythagorean Theorem (E)
▶️ Adding and subtracting polynomials (A)
▶️ Adding and subtracting polynomials (B)
▶️ Multiplying Polynomials - Basic (A)
▶️ Multiplying Polynomials - Basic (B)
▶️ Multiplying Polynomials - Intermediate (A)
▶️ Multiplying Polynomials - Intermediate (B)
▶️ Multiplying Polynomials - Advanced (A)
▶️ Multiplying Polynomials - Advanced (B)
▶️ Extended Practice: Dividing Polynomials
▶️ Factoring the difference of two squares (A)
▶️ Factoring the difference of two squares (B)
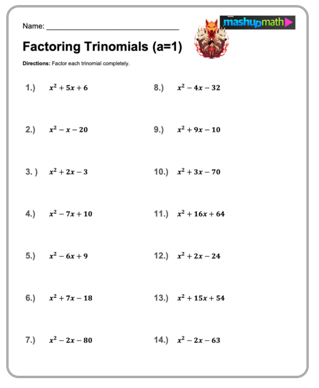
▶️ Factoring Trinomials (a=1) (A)
▶️ Factoring Trinomials (a=1) (B)
▶️ Extended Practice: Factoring Trinomials (a=1)
▶️ Factoring Trinomials (a>1) (A)
▶️ Factoring Trinomials (a>1) (B)
▶️ Extended Practice: Factoring Trinomials (a>1)
▶️ Factoring/Solving Trinomials by Completing the Square (A)
▶️ Factoring/Solving Trinomials by Completing the Square (B)
▶️ Translating variable expressions into words (A)
▶️ Translating variable expressions into words (B)
▶️ Translating variable equations into words (A)
▶️ Translating variable equations into words (B)
▶️ Writing algebraic expressions (one step) (A)
▶️ Writing algebraic expressions (one step) (B)
▶️ Writing algebraic expressions (one step) (C)
▶️ Writing algebraic expressions (one or two step) (A)
▶️ Writing algebraic expressions (one or two step) (B)
▶️ Writing algebraic expressions (one or two step) (C)
▶️ Evaluating expressions (add/subtract) (one variable) (A)
▶️ Evaluating expressions (add/subtract) (one variable) (B)
▶️ Evaluating expressions (multiply/divide) (one variable) (A)
▶️ Evaluating expressions (multiply/divide) (one variable) (B)
▶️ Evaluating expressions (4 operations) (one variable) (A)
▶️ Evaluating expressions (4 operations) (one variable) (B)
▶️ Evaluating expressions (w/ exponents) (one variable) (A)
▶️ Evaluating expressions (w/ exponents) (one variable) (B)
▶️ Evaluating expressions (add/subtract) (two variables) (A)
▶️ Evaluating expressions (add/subtract) (two variables) (B)
▶️ Evaluating expressions (multiply/divide) (two variables) (A)
▶️ Evaluating expressions (multiply/divide) (two variables) (B)
▶️ Evaluating expressions (4 operations) (two variables) (A)
▶️ Evaluating expressions (4 operations) (two variables) (B)
▶️ Evaluating expressions (w/ exponents) (two variables) (A)
▶️ Evaluating expressions (w/ exponents) (two variables) (B)
▶️ Simplifying expressions (combine like terms) (A)
▶️ Simplifying expressions (combine like terms) (B)
▶️ Simplifying expressions (combine like terms) (C)
▶️ Solving one-step algebraic equations (add/subtract) (A)
▶️ Solving one-step algebraic equations (add/subtract) (B)
▶️ Solving one-step algebraic equations (multiply/divide) (A)
▶️ Solving one-step algebraic equations (multiply/divide) (B)
▶️ Solving one-step algebraic equations (4 operations) (A)
▶️ Solving one-step algebraic equations (4 operations) (B)
▶️ Solving one-step algebraic equations (4 operations) (C)
▶️ Extended Practice: Solving One-Step Equations (A)
▶️ Extended Practice: Solving One-Step Equations (B)
▶️ Solving Two-Step Algebraic Equations (A)
▶️ Solving Two-Step Algebraic Equations (B)
▶️ Solving Two-Step Algebraic Equations (C)
▶️ Solving Two-Step Equations Extended Practice (A)
▶️ Solving Two-Step Equations Extended Practice (B)
▶️ Solving 2-sided algebraic equations (A)
▶️ Solving 2-sided algebraic equations (B)
▶️ Solving 2-sided algebraic equations (C)
▶️ Solving Multi-Step Equations Extended Practice (Beginner)
▶️ Solving Multi-Step Equations Extended Practice (Intermediate)
▶️ Solving Multi-Step Equations Extended Practice (Advanced)
▶️ Completing two variable equation tables (A)
▶️ Completing two variable equation tables (B)
▶️ Completing two variable equation tables (C)
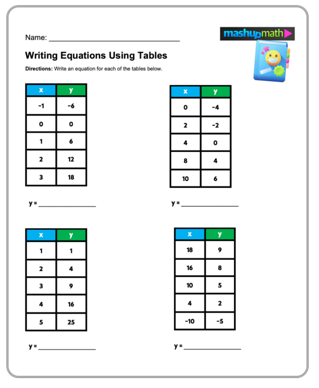
▶️ Writing equations using tables (A)
▶️ Writing equations using tables (B)
▶️ Writing equations using tables (C)
▶️ Exploring Patterns with X-Y Equation Tables
▶️ Solving multi-step equations (A)
▶️ Solving multi-step equations (B)
▶️ Solving multi-step equations (C)
▶️ Solving absolute value equations (A)
▶️ Solving absolute value equations (B)
▶️ Parallel Lines Cut by a Transversal: Classifying (A)
▶️ Parallel Lines Cut by a Transversal: Classifying (B)
▶️ Parallel Lines Cut by a Transversal: True or False? (A)
▶️ Parallel Lines Cut by a Transversal: True or False? (B)
▶️ Parallel Lines Cut by a Transversal: 1 Missing Angle (A)
▶️ Parallel Lines Cut by a Transversal: 1 Missing Angle (B)
▶️ Parallel Lines Cut by a Transversal: 2 Missing Angles (A)
▶️ Parallel Lines Cut by a Transversal: 2 Missing Angles (B)
▶️ Graphing single-variable inequalities on a number line (A)
▶️ Graphing single-variable inequalities on a number line (B)
▶️ Solving one-step inequalities (A)
▶️ Solving one-step inequalities (B)
▶️ Solving two-step inequalities (A)
▶️ Solving two-step inequalities (B)
▶️ Solving multi-step inequalities (A)
▶️ Solving multi-step inequalities (B)
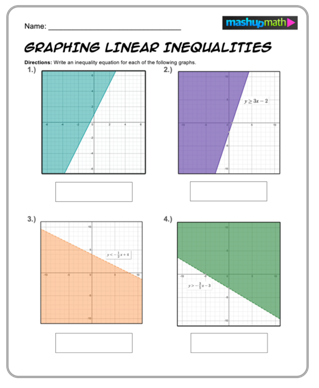
▶️ Graphing linear inequalities on the coordinate plane (A)
▶️ Finding slope from a graph (A)
▶️ Finding slope from a graph (B)
▶️ Finding slope using a formula (A)
▶️ Finding slope using a formula (B)
▶️ Finding slope from an equation (A)
▶️ Finding slope from an equation (B)
▶️ Practice with Slope-Intercept Form (A)
▶️ Practice with Slope-Intercept Form (B)
▶️ Practice with Slope-Intercept Form (C)
▶️ Graphing lines in slope-intercept form (A)
▶️ Graphing lines in slope-intercept form (B)
▶️ Graphing lines in slope-intercept form (C)
▶️ Practice Writing Linear Equations (A)
▶️ Practice Writing Linear Equations (B)
▶️ Classifying parallel and perpendicular lines (A)
▶️ Classifying parallel and perpendicular lines (B)
▶️ Practice with Linear Inequalities on the Coordinate Plane (A)
▶️ Practice with Linear Inequalities on the Coordinate Plane (B)
▶️ Practice with Linear Inequalities on the Coordinate Plane (C)
▶️ Practice with Linear Inequalities on the Coordinate Plane (D)
▶️ Practice with Linear Inequalities on the Coordinate Plane (E)
▶️ Graphing absolute value functions (A)
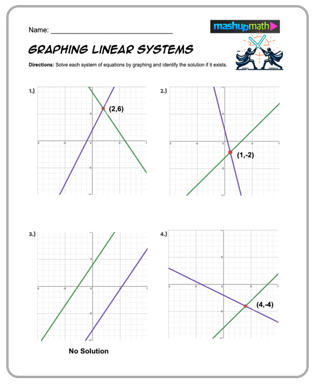
▶️ Solving linear systems of equations by graphing (A)
▶️ Solving linear systems of equations by graphing (B)
▶️ Solving linear systems of equations by graphing (C)
▶️ Solving linear systems of equations by graphing (D)
▶️ Solving linear systems of equations by graphing (E)
▶️ Solving systems of linear inequalities by graphing (A)
▶️ Solving systems of linear inequalities by graphing (B)
▶️ Solving systems of linear inequalities by graphing (C)
▶️ Solving Quadratic Functions by Factoring (A)
▶️ Solving Quadratic Functions by Factoring (B)
▶️ Solving Quadratic Functions by Factoring (C)
▶️ Solving Quadratic Functions by Completing the Square (A)
▶️ Solving Quadratic Functions by Completing the Square (B)
▶️ Solving Quadratic Functions by Completing the Square (C)
▶️ Graphing Quadratic Functions (A)
▶️ Graphing Quadratic Functions (B)
▶️ Graphing Quadratic Functions (C)
▶️ Quadratic Formula Practice (A)
▶️ Practice with Mean, Median, and Mode (A)
▶️ Practice with Mean, Median, and Mode (B)
▶️ Drawing Double Line Graphs (A)
▶️ Drawing Double Line Graphs (B)
▶️ Analyzing Double Line Graphs (A)
▶️ Analyzing Double Line Graphs (B)
▶️ Analyzing Double Line Graphs (C)
▶️ Making Box-and-Whisker Plots (A)
▶️ Making Box-and-Whisker Plots (B)
▶️ Making Box-and-Whisker Plots (C)
▶️ Analyzing Box-and-Whisker Plots (A)
▶️ Analyzing Box-and-Whisker Plots (B)
▶️ Analyzing Box-and-Whisker Plots (C)
▶️ Modeling situations using linear equations word problems (A)
▶️ Modeling situations using linear equations word problems (B)
▶️ Variables and expressions word problems (A)
▶️ Variables and expressions word problems (B)
▶️ Variables and expressions word problems (C)
▶️ Variables and equations word problems (A)
▶️ Variables and equations word problems (B)
▶️ Variables and equations word problems (C)
▶️ Solving One-Step and Two-Step Equations Word Problems (A)
▶️ Solving One-Step and Two-Step Equations Word Problems (B)
▶️ Scientific Notation Word Problems (A)
▶️ Scientific Notation Word Problems (B)
▶️ GCF and LCM Word Problems (A)
▶️ GCF and LCM Word Problems (B)
▶️ Proportion word problems (A)
▶️ Proportion word problems (B)
▶️ Pythagorean Theorem word problems (A)
▶️ Pythagorean Theorem word problems (B)
▶️ Pythagorean Theorem word problems (C)
▶️ Distance, rate, and time word problems (A)
▶️ Distance, rate, and time word problems (B)
▶️ Multi-step Word Problem: Snappy Rental Car
▶️ Multi-step Word Problem: Neil’s Square Paper
▶️ Multi-step Word Problem: Lilly Goes Shopping
▶️ Multi-step Word Problem: Pumpkins and Watermelons
▶️ Multi-step Word Problem: Alejandro’s Rock Collection
Can’t find what you’re looking for? Have a request? Found a typo or a mistake? Send us a message with Worksheets in the subject line.