Calculating Percent Change in 3 Easy Steps

Learning how to calculate percent change is an immensely handy and essential mathematical skill that has various applications inside of your classroom and in the real world as well.

(Looking for a Percent Change Calculator to make a super fast calculation: Click here to access our free Percent Change Calculator)

The ability to calculate, comprehend, and analyze percentages does not only come in handy on class assignments, assessments, quizzes, exams, the SAT, etc., but also in practical matters associated with every day life and several careers as well. In fact, percentages is one of the most useful math skills outside of the classroom, so understanding them is should not be discounted or overlooked.

While it is common for students to think that calculating percent change can be a confusing and demanding task with many steps, the reality is that correctly solving any percent change problem is actually extremely simple and painless.

Are you ready to learn how to easily solve any percent change problem? This post shares a free Calculating Percent Change step-by-step lesson guide that will painlessly teach you how to calculate percent change using an uncomplicated three-step process. If you can learn how to follow the three-step process demonstrated in this guide, you will gain the ability to swiftly and flawlessly solve any math problems or exercises that require you to find percent change.

Now that we are just about ready to learn the three-step process for calculating percent change, lets quickly review a handful of essential vocabulary words, concepts, and definitions related to percentages and percent change.

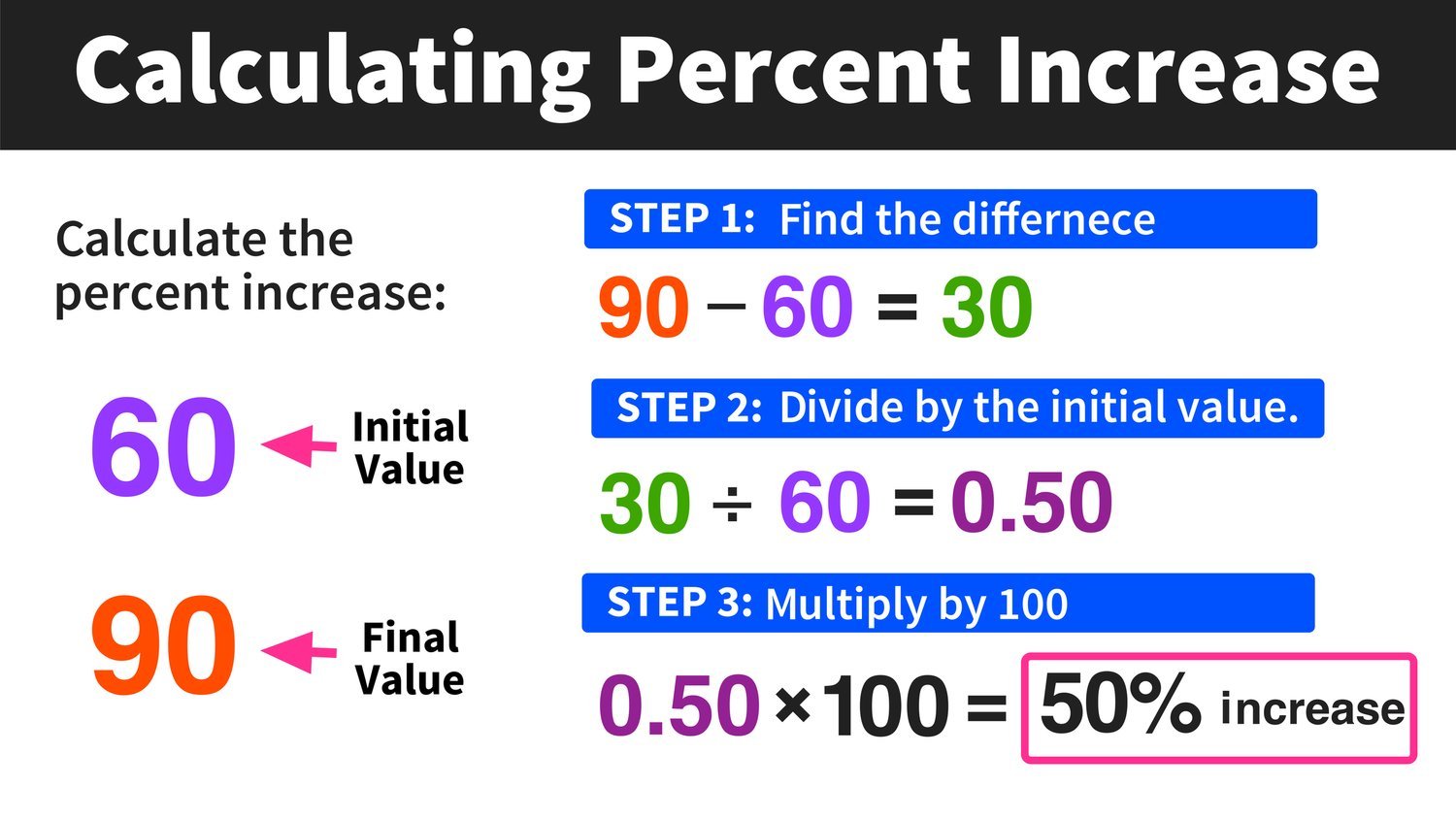
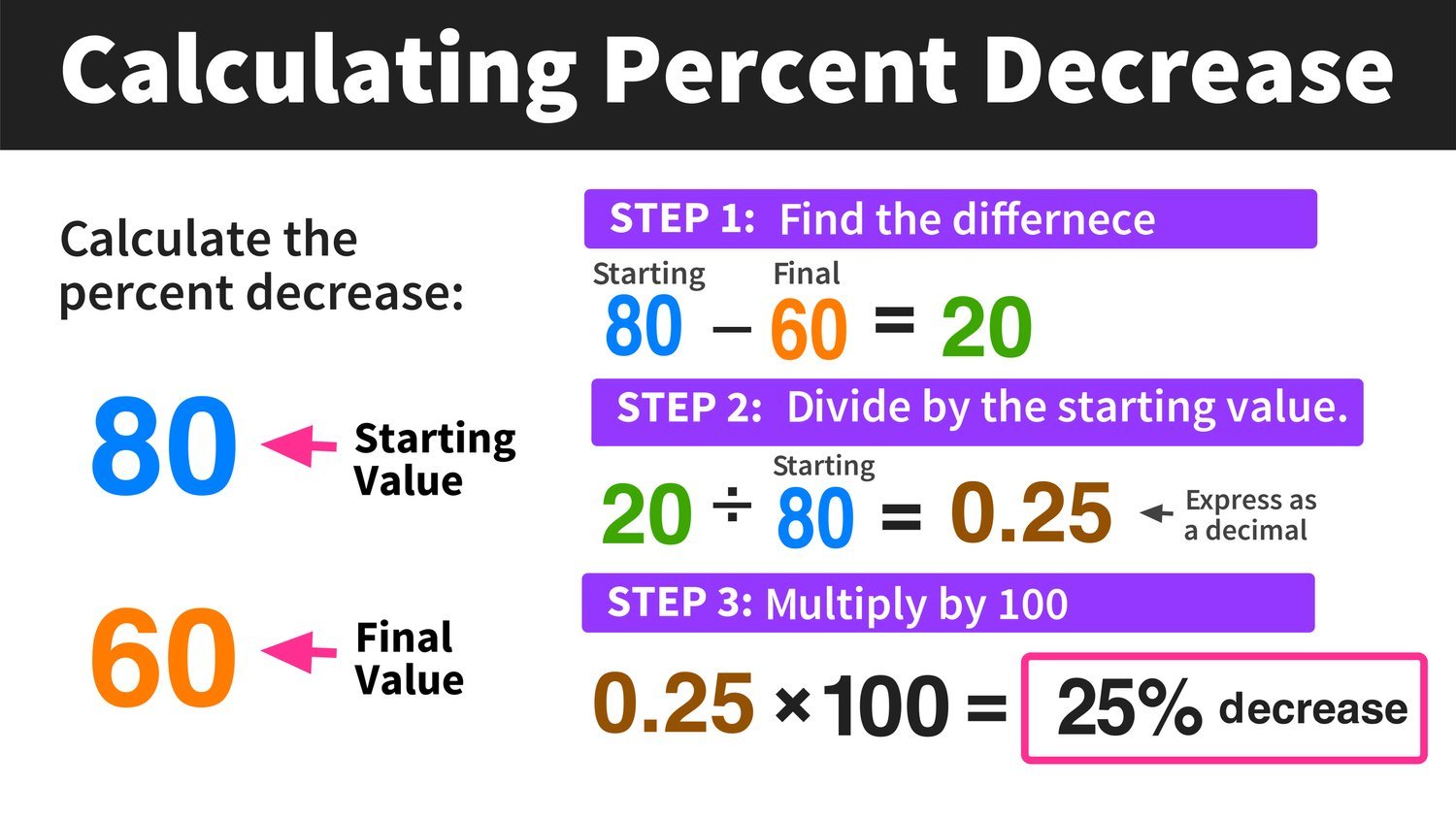
Looking to learn how to calculate percent increase or percent decrease? Use the links below to download our free step-by-step guides:

Percent Definition

In terms of numeracy and mathematics, the word percent relates to parts per one hundred and the numerical symbol used to express percentages is %.

A simple example of a percent would be the value 60%. In this case, 60% represents 60 per 100.

Take a look at the diagram below. You should notice that the green shaded region makes up 60% of the whole.

Another way to express percent is to say that it is defined as a ratio of a value out of one hundred.

For another simple example, consider 30%. In this case, 30% is defined as 30 out of every 100. When viewing percentages as ratios of values out of one hundred, you can conclude that if 30% of 500 total people ride the train to work, then 150 total people ride the train—since 30 out of every 100 means that for every group of 100, we have to consider 30 people. So, 30 x 5 = 150 and 30% of 500 is 150.

Absolute Value Definition

To correctly calculate percent change, you will have to use absolute values. So, let’s do a quick review before we move on.

In math, the absolute value of a real number x, denoted using the vertical brackets |x|, is the positive (or non-negative) value of x.

The key thing to understand is that absolute values are always positive.

For example, the absolute value of 25 is equal to positive 25 AND the absolute value of -25 is equal to positive 25.

|25| = 25
|-25| = 25

This fact also applies to expressions inside of absolute value bars, for example:

| 5 - 7 | = | -2 | = 2
| 88 - 10 | = | 78 | = 78
| 0 - 33 | = | -33 | = 33

Again, notice how the result is always positive.

Percent Change Definition

Now you are ready to consider the question: what is percent change?

In math, the percent change between two values—a starting value and a final value—is simply the difference between those two values expressed as a percentage.

Note that percent change can also be referred to in terms of percent increase or percent decrease. These terms are more specific than percent change.

Percent Increase means that the final value is larger than the starting value. Final Value > Starting Value
Percent Decrease means that the final value is smaller than the starting value. Final Value < Starting Value

Note that percent change will be expressed as a number with a percentage (%) symbol attached to it.

As for identifying the starting value and the final value, simply look for the first value that is given and the second value that is given (percent change problems will always involve two values).

For example, if Chris drove 120 miles in September and 150 miles in October, and you wanted to calculate the percent change in miles driven between the two months, you would start by identifying that the starting value is 120 and the final value is 150.

Simple enough? If not, please review the previous section again as being able to correctly identify the starting value and the final value is critical for finding the solution to percent change math problems.

Calculating Percent Change

Are you ready to learn how to calculate percent change by apply our easy three-step process?

For our first example, we will continue with the scenario involving Chris and his monthly driving mileage totals.

Calculating Percent Change Example #1

In our first example, we will calculate the percent change for the following situation:

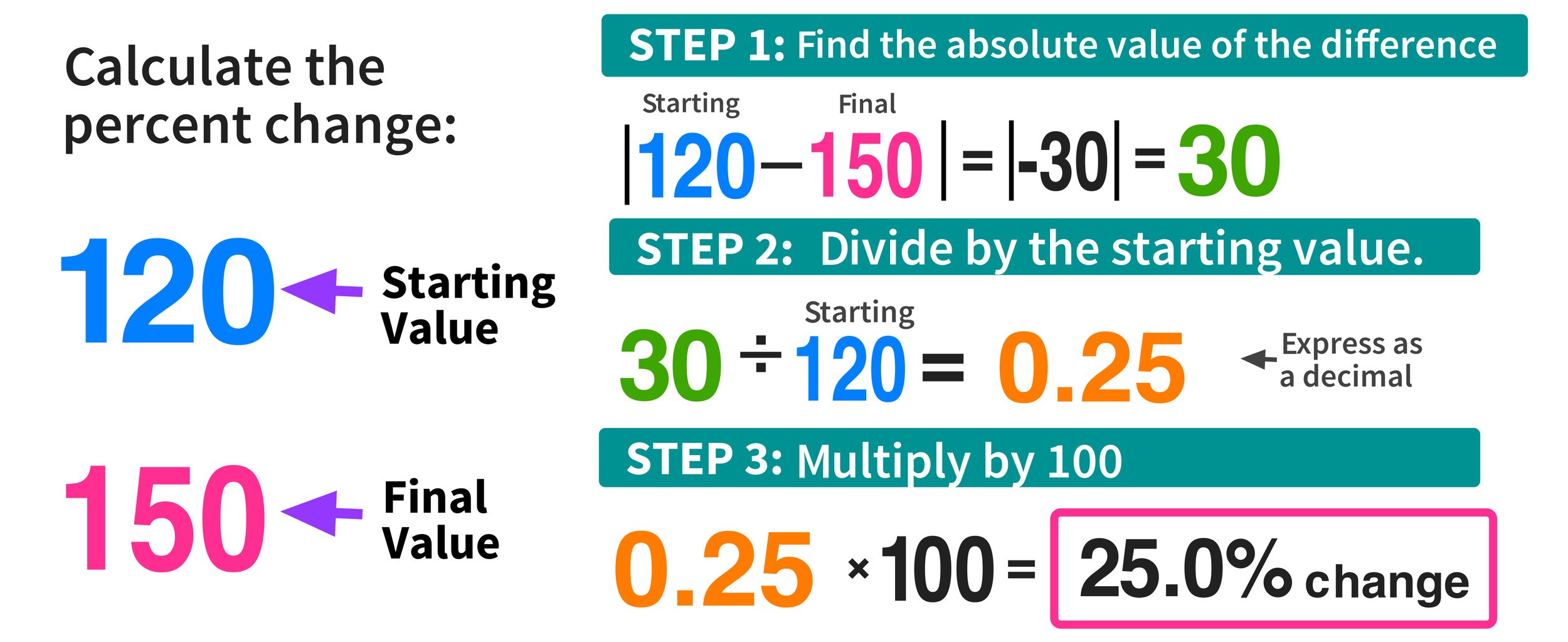
Chris drove 120 miles in September and 150 miles in October. What is the percent change in miles driven between the two months?

Remember that you already figured out that the starting value is 120 and the final value is 150.

Now, you are ready to apply the three-step process to calculating percent change. Here is a preview of how it will work:

Step 1: Find the absolute value of the difference.

To perform step one, simply take the absolute value of the difference of the starting value and the final value.

| Starting Value - Final Value | = ?

In this example:

| 120 - 150 | = | -30 | = 30

Again, since absolute value is involved, the end result of step 1 will always be a positive number.

Step 2: Divide the difference by the starting value.

The second step is to take your result from step 1 (30 in this example) and divide it by the starting value (120 in this example) as follows:

30/120 = 0.25

For the second step, you will always express your result as decimal (never as a fraction). Otherwise, you will not be able to perform the third and final step.

Step 3: Multiply by 100

The last step is easy and straightforward: take your decimal result from step 2 and multiply it by 100, then express your final result using a % symbol as follows:

0.25 x 100 = 25.0%

Final Answer: 25% Change (or 25% Increase)

Note that this final answer can also be considered a percent increase since the final value was larger than the starting value (the number of miles driven increased over time).

That’s all there is to it! Applying the three-step process allows you to conclude that there was a 25% change in miles driven between September and October.

Now, let's take a look at another calculating percent change example problem where you will gain more practice applying the three-step process.

Looking for a free Percent Change Calculator?

If you need a faster way to calculate the percent change between two numbers, check out our free Percent Change Calculator tool, which lets you input the starting and final values to get an instant answer!

▶ Click here to access our free Percent Change Calculator for students

Calculating Percent Increase Example #2

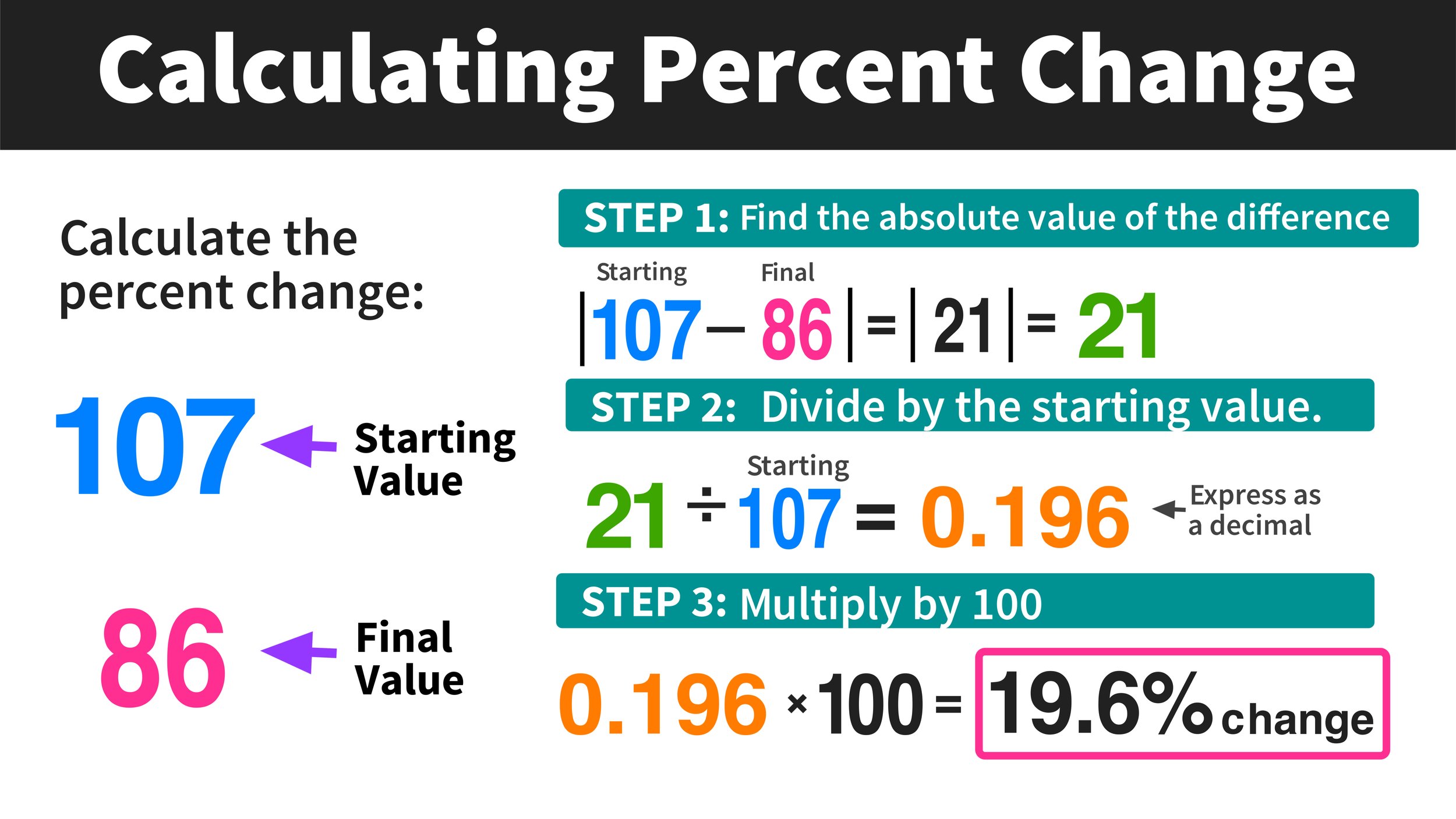
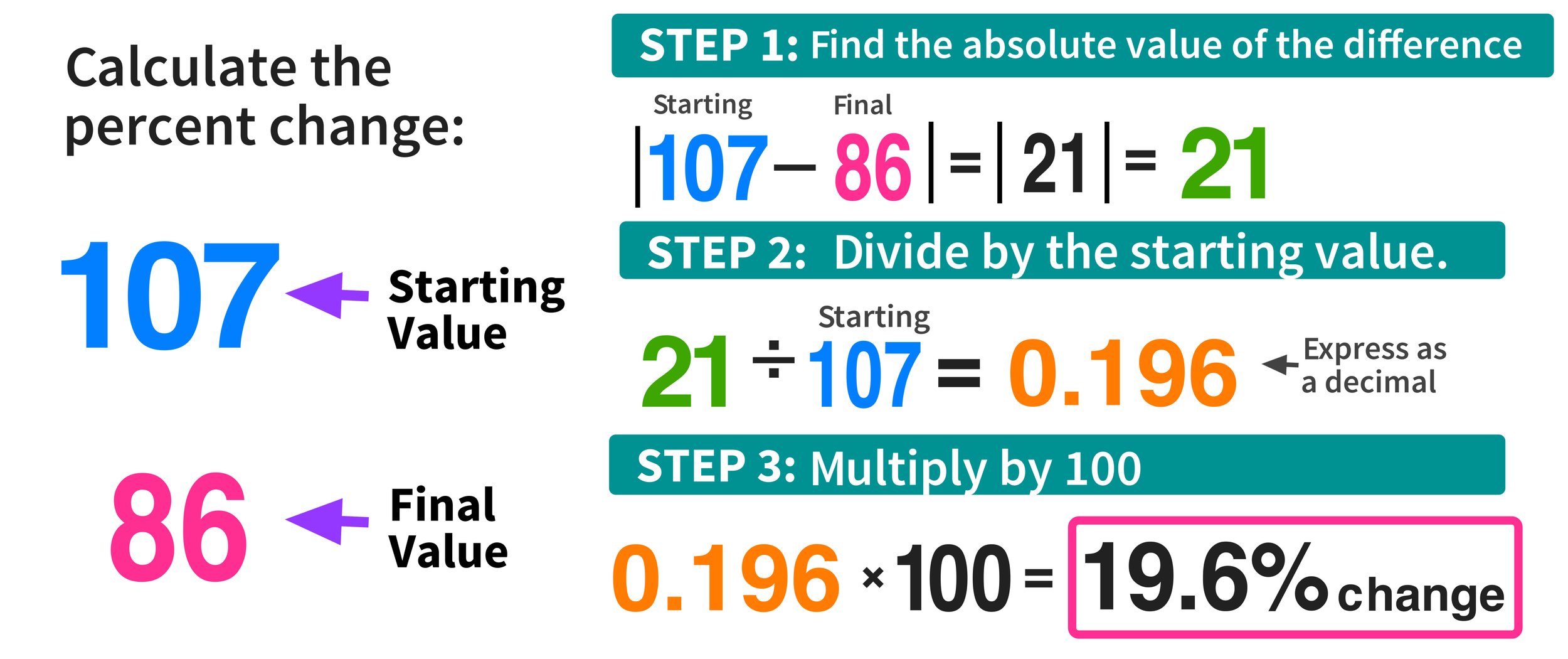
Last semester, Ariana spent a total of 107 hours studying for exams. This semester, she spent a total of 86 hours studying for exams. What was the percent change in the total number of hours she spent studying between last semester and this semester?

Let’s start by identifying the starting value and the final value:

Starting Value: 107
Final Value: 86

Step 1: Find the absolute value of the difference.

Just like Example #1, start by finding the absolute value of the difference of the starting value and the final value.

| 107 - 86 | = | 21 | = 21

Step 2: Divide the difference by the starting value.

The next step is to take your result from step 1 (21 in this example) and divide it by the starting value (107 in this example) as follows:

21/107 = 0.1962616822… ≈ 0.196

Remember to express your result as decimal!

Step 3: Multiply by 100

Finally, take your decimal result from step 2 and multiply it by 100, then express your final result using a % symbol as follows:

0.196 x 100 = 19.6%

Final Answer: 19.6% Change (or 19.6% Decrease)

Note that this final answer can also be considered a percent decrease since the final value was smaller than the starting value (the number of hours spent studying decreased over time).

All done! You have concluded that there was a 19.6% change in the total hours Ariana spent studying between last semester and this semester.

Now, let’s gain some more experience using the three-step process for calculating percent change by working through one final example.

Calculating Percent Decrease Example #3

In 2023, 395 students attended the Loha High School Dance. In 2024, 861 students attended the Loha High School Dance. What was the percent change in the number of students who attended the Loha High School Dance between 2023 and 2024?

We will start example #3 the same as the previous two examples, by identifying the starting value and the final value:

Starting Value: 395
Final Value: 861

Just like the last two examples, you can solve this problem by following the three-step process:

Step 1: Find the absolute value of the difference.

Start by finding the absolute value of the difference of the starting value and the final value.

| 395 - 861 | = | -466 | = 466

Step 2: Divide the difference by the starting value.

The next step is to take your result from step 1 (466 in this example) and divide it by the starting value (395 in this example) as follows:

466/395 = 1.179746835… ≈ 1.18

Step 3: Multiply by 100

Finally, take your decimal result from step 2 and multiply it by 100, then express your final result using a % symbol as follows:

1.18 x 100 = 118.0%

Final Answer: 118% Change (or 118% Increase)

Note that this final answer can also be considered a percent increase since the final value was larger than the starting value (the number students in attendance increased over time).

Also note that it is totally fine to have an end result that is greater than 100%. In this case, a 118% increase means that the number of students in attendance more than doubled!

After working through three percent change examples, you should be feeling more confident in your ability to solve percent change problems using the three-step process. However, I highly recommend working through the examples again to further solidify your understanding so that you are successful on problems.

Conclusion: Calculating Percent Change

You can calculate percent change using a given starting value and final value by applying the following 3-step process:

Step 1: Find the absolute value of the difference between the starting value and the final value.

Step 2: Divide the result from Step 1 by the starting value and always express the result in decimal form.

Step 3: Multiply the result from Step 2 by 100 and express your final answer as a percentage (%).

What about Calculating Percent Increase and Percent Decrease?

Learn how to calculate a percent increase or a percent decrease between two numbers using our free step-by-step guides. Click the links below to get started.