What is Point-Slope Form?

Arranging equations in a particular format gives us insight into specific features of an equation. The point-slope form is one such form used with linear equations and is useful when building an equation of a given straight line.

Let’s walk through what the point-slope form is, and learn its use cases with examples.

By the end of this lesson guide, you will be able to construct linear equations in the point-slope form and interpret features like slope, y-intercepts, and x-intercepts.

What are the different forms of linear equations?

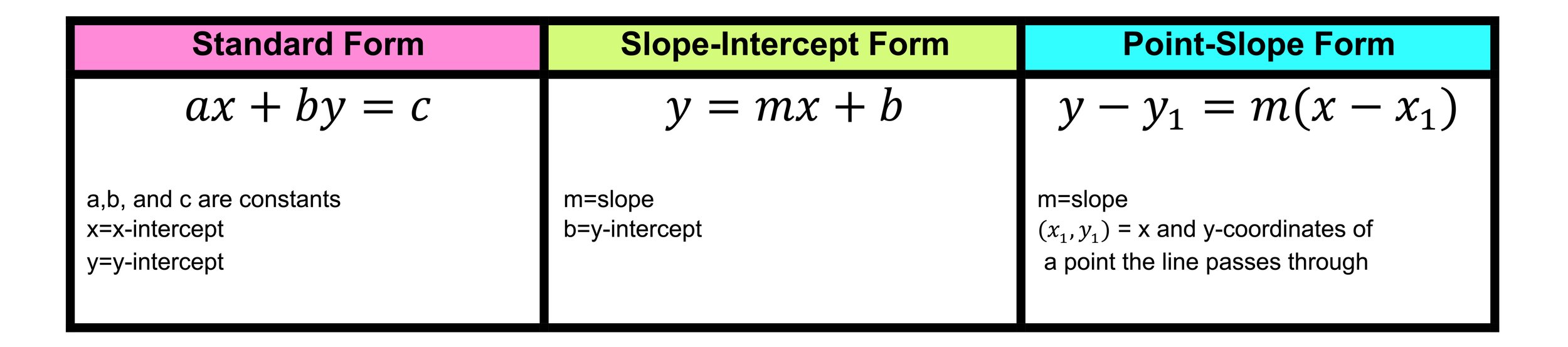
A linear equation or an equation of a straight line can be represented in three main forms. These forms are namely:

Standard form
Slope-intercept form
Point-slope form

The major differences between these forms are the way that the properties of a linear equation are presented within the equation. The key properties that are involved in a straight line are the slope and its intercepts (y-intercept and/or x-intercept).

The standard form, also known as the general form of linear equations, is Ax + By = C, where A, B, and C are constants. Using the standard form to represent a straight line makes it easier to obtain the y and x-intercepts using substitution.

The slope-intercept form is the most commonly used form and is of the form y = mx + b, where m and b are constants. The benefit of using the slope-intercept is that the slope and y-intercept of the straight line can be obtained directly by observing the equation, as m is the slope, and b is the y-intercept.

What is Point-Slope Form?

The point-slope form is another form in which a linear equation with two variables can be represented. As the name suggests, to construct an equation in the point-slope form we require a point on the straight line and its slope.

Definition: The point-slope form of a line is expressed using the slope of the line and point that the line passes through.

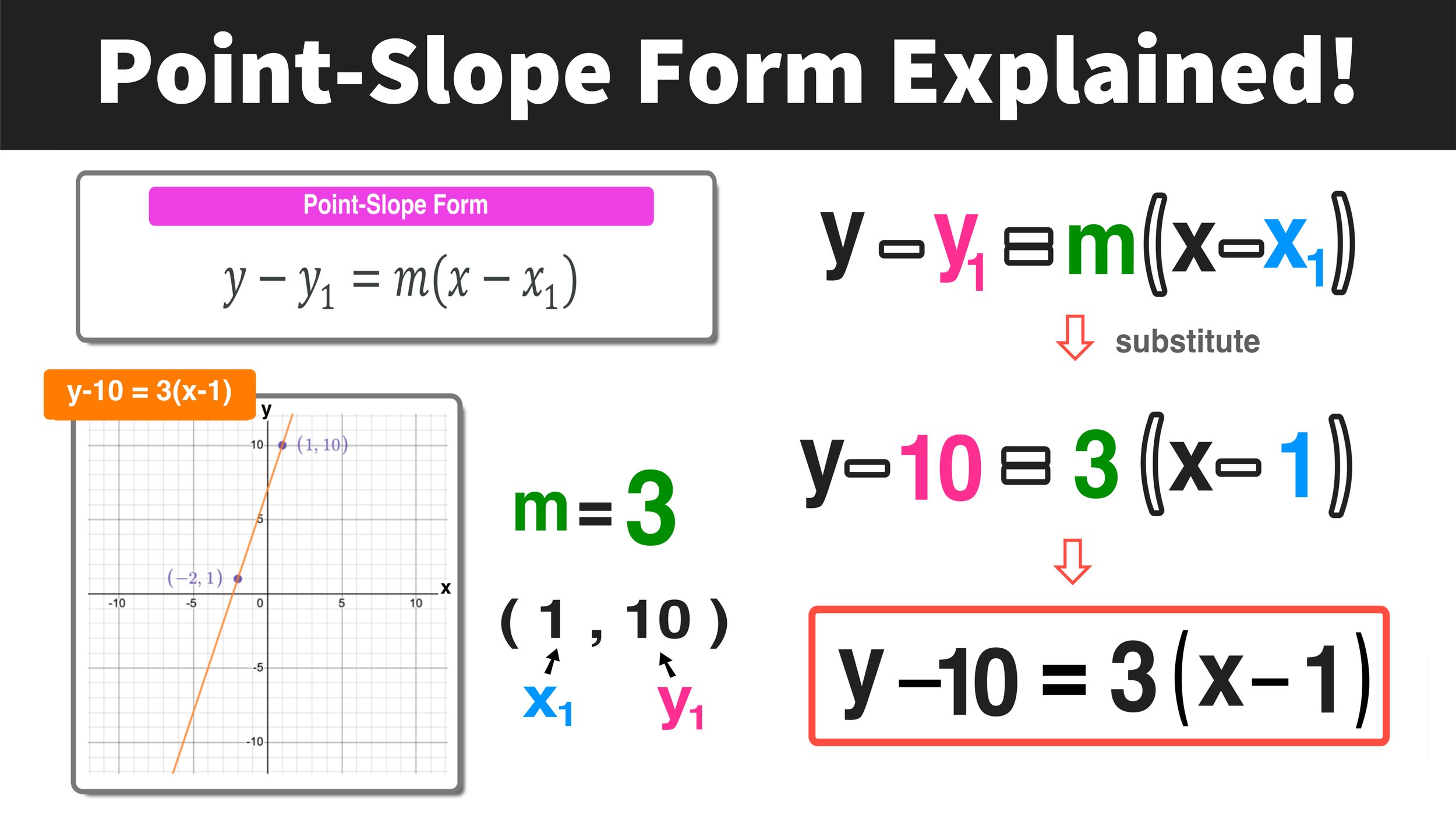
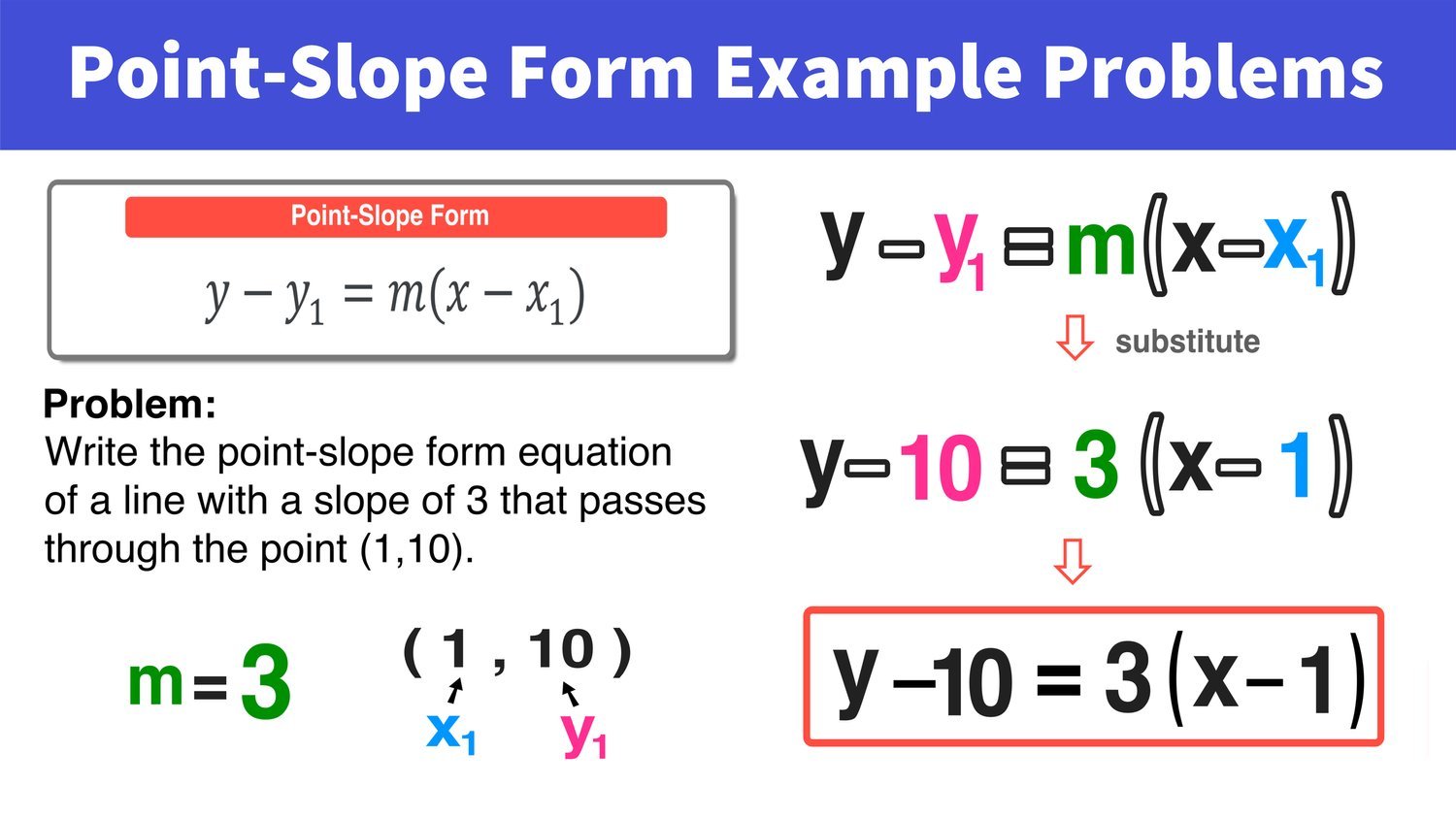
Formula: y-y1 = m(x - x1) where m equals the slope of the line and x1 and y1 equal the corresponding x- and y-coordinates of a given point the line passes through.

The general structure of an equation in the point-slope form is: y - y1 = m*(x - x1)

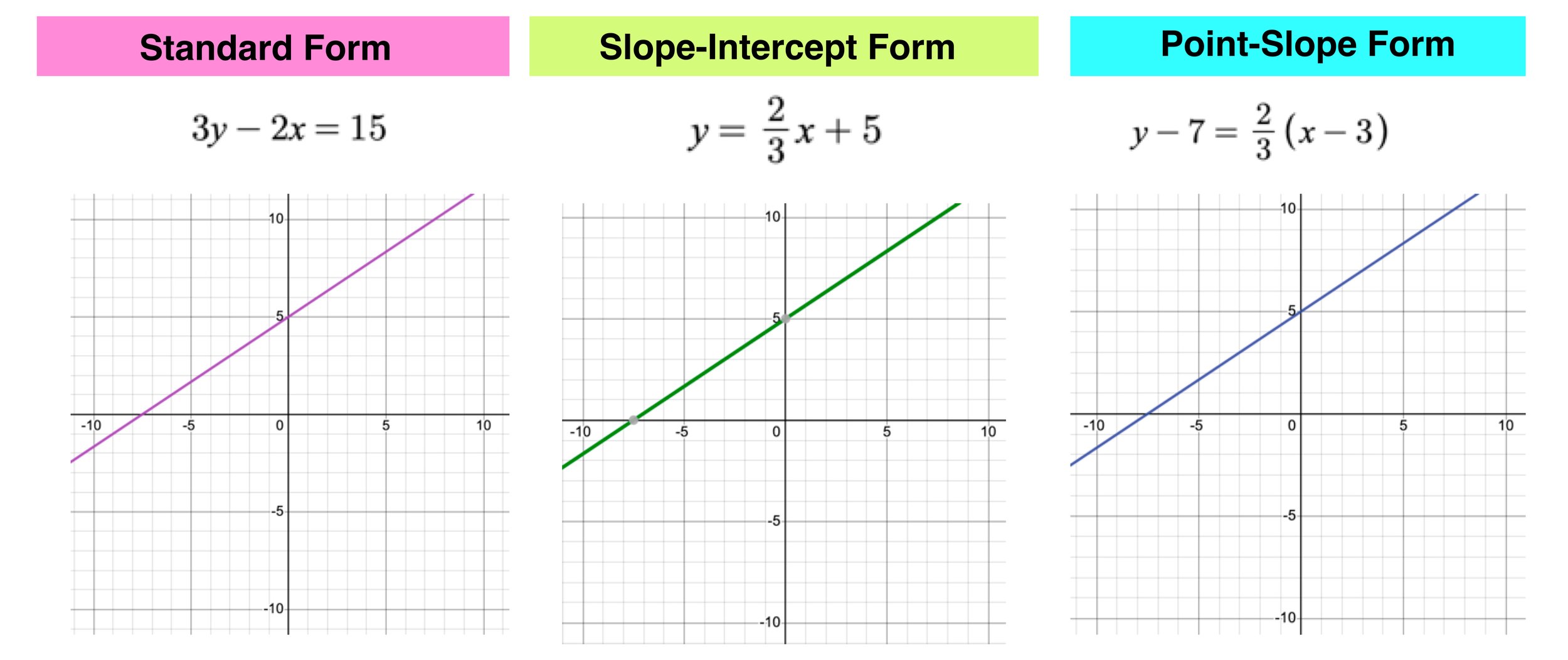
Here (x1,y1) is any arbitrary point on the straight line, and m is the slope/gradient of the straight line. Following is a table that shows a side-by-side comparison of the same straight line represented in the three forms mentioned above.

Again, notice each equation is a different way of expressing the same linear function, namely one with a slope of 2/3 and a y-intercept of 5.

One of the unique properties of the point-slope form compared to the other forms is the flexibility in its structure. This flexibility comes from the fact that you can use any point on a particular straight line to build the point-slope form equation.

In fact, below are some out of infinitely many other valid point-form equations of the straight line above.

y - 9 = ⅔*(x-6)
y - 3 = ⅔*(x+3)
y +1 = ⅔*(x+9)

Next, let’s find out more about the fundamentals behind the point-slope form.

Fundamentals of the point-slope form

The main concept behind the point-slope form is the formula for determining the slope of a straight line. The formula that is used to calculate the slope of a straight line is :

m = (y2 - y1)/(x2 - x1)

Here, m is the slope of the straight line, while (x1,y1) and (x2, y2) are the coordinates of any two points on the given straight line.

Let’s apply the slope formula to find the slope of the line given in the graph below:

(y - y1)/ (x - x1) = m

Multiply both sides by x - x1, and we get

y - y1 = m * (x-x1), which is the general structure of the point-slope form.

As mentioned before, we can replace (x1,y1) with the coordinates of any point that lies on the given straight line. Note that if you select the y-intercept (b,0), then you will end up with the slope-intercept form of the equation.

y - b = m*(x-0)

y - b = mx

y = mx + b → the slope-intercept form

Therefore, the slope intercept can be considered a special case of the point-slope form.

Now with this fundamental understanding let’s see how we can construct the equation of a straight line in point-slope form using a variety of examples.

Point-Slope Form Examples

The information provided in a scenario can be different, but the goal is to determine the slope and the coordinates of a point that lies on a straight line.

Example #1 Finding the point-slope equation from slope and a point

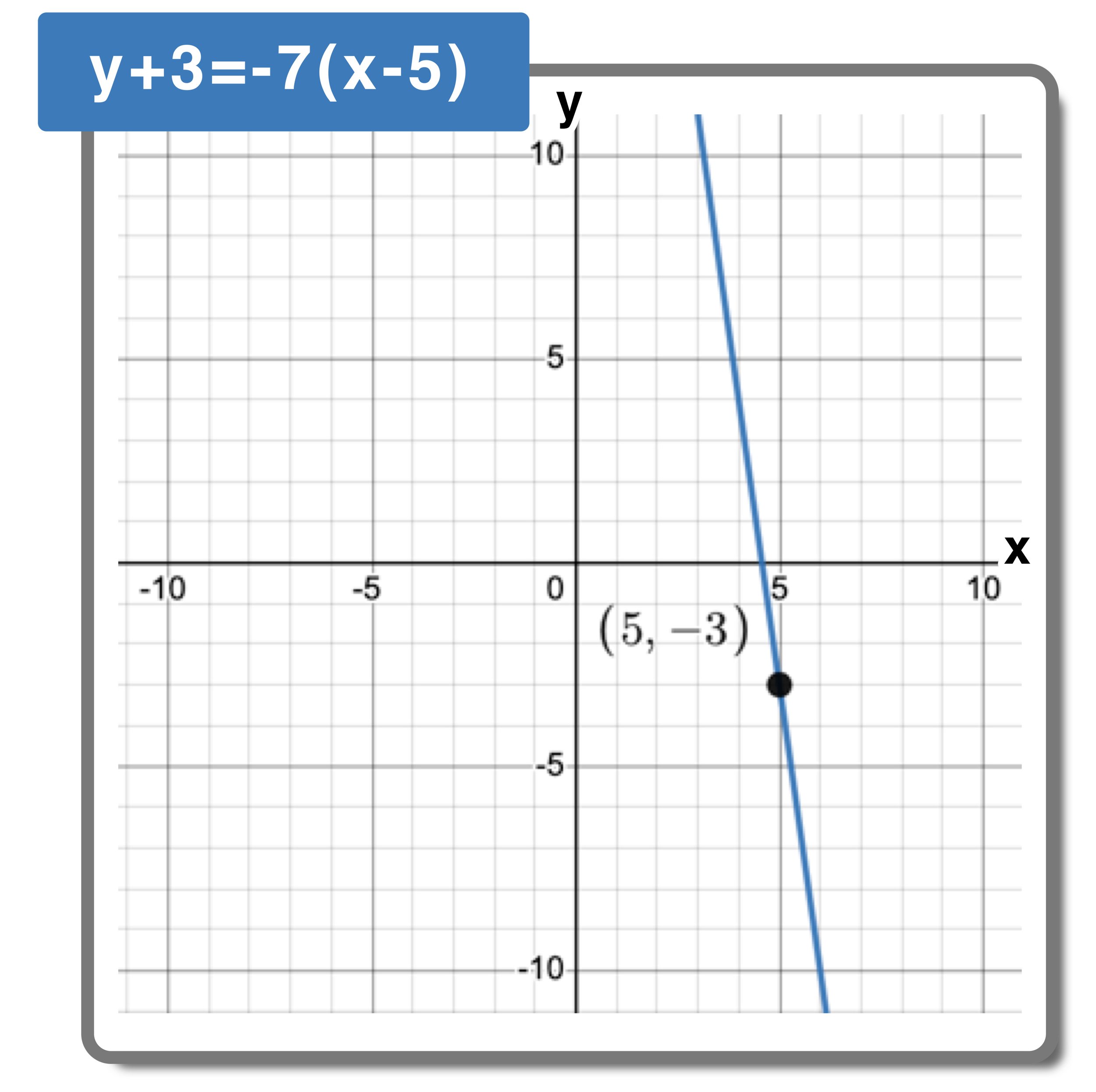
Problem: Consider a straight line that has a slope of -7 and passes through the point (5, -3)

We can directly substitute the given data into the point-slope general equation below:

y-y1 = m*(x-x1)

Here m is the slope, hence it is -7 in this example. The point (5,3) is the arbitrary point (x1,y1). By substituting the terms we get:

y - - 3 = -7*(x - 5)

Hence,

y + 3 = -7*(x - 5) is the point-form equation of the straight line.

Example #2 Finding point-slope equation from two points

Problem: Consider a straight line on which the points (-2,1) and (1,10) lie. Determine the equation of the line in point-slope form.

First, we can use the slope formula to determine m (slope) of the line.

m = (y2- y1)/(x2-x1)

Substituting (-2,1) and (1,10) we get:

m = (10-1)/(1- - 2)

m = 9/3

m = 3

Now that we have determined the slope of the line to be 3, we can build the point-slope equation.

y-y1 = m*(x-x1)

We can pick any point on the line for (x1,y1). Here let’s use (-2,1).

y - 1 =3*(x- -2)

Hence,

y - 1 = 3*(x+2) is the point-form equation of the straight line.

Example #3 Determining features from the point-slope equation

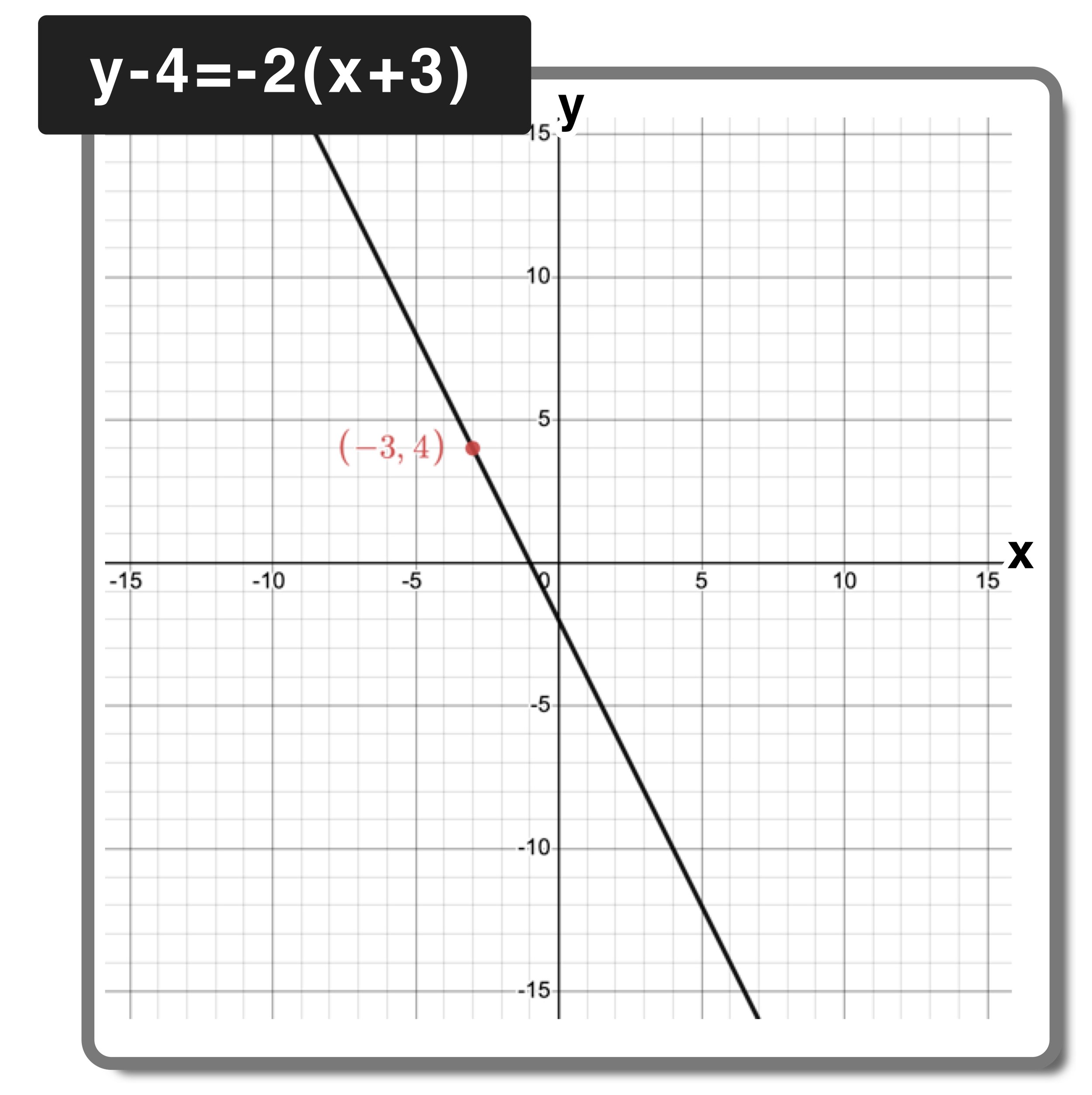
Problem: Which of the following graphs represents the straight line of equation y - 4 = -2(x + 3)

The equation provided is in the point-slope form. By comparing y - 4 = -2(x+3) and y - y1 = m*(x-x1), we can work out that:

m = -2, and (x1,y1) is (4,-3)

So we must look for a graph that has a slope of -2 and passes through (4,-3). Since only black, red, and blue lines clearly pass through (4,-3), we can eliminate the rest.

Amongst the three, only the black has a negative slope. Hence the line that represents the point-slope equation of y - 4 = -2(x+3) is the black graph.

Looking for more point-slope form example problems?

Conclusion: Point-Slope Form

Amongst the three main forms of representing linear equations, the point-slope form can be considered the most versatile. By identifying the slope and a single point that lies on a straight line you can determine the point-slope of an equation. The type of information you get about a straight line may change, hence you should utilize the necessary formulas and/or visual interpretations of graphs to extract what you need.