The PEMDAS Rule Explained!

What is the PEMDAS Rule and how does it apply to the math order of operations?

What is the PEMDAS rule for math order of operations and solving problems? When studying math, you learn about a process called the order of operations. This process is a rule that must be followed when solving math problems that have multiple operations such as subtraction, addition, multiplication, division, groupings, and/or exponents.

There are many memory tricks for remembering the math order of operations in the correct order, but the most popular is the PEMDAS rule.

The PEMDAS Rule is a mnemonic that stands for:

P: Parenthesis

E: Exponents

M: Multiplying

D: Dividing

A: Adding

S=Subtracting

The operations included in the PEMDAS rule are performed left to right.

Additionally, the PEMDAS rule for recalling the math order of operations has a few important sub-rules that must also be followed if you want to use PEMDAS correctly (and get the correct answers to math problems). These important sub-rules relate to the relationships between multiplying/dividing and adding/subtracting.

These important PEMDAS rule sub-rules are explained in detail in the next section:

PEMDAS Rule: Key Points

The PEMDAS rule has been around for decades as a tool for helping students to remember the math order of operations. Many prefer to simply remember the mnemonic PEMDAS (pronounced PEM-DAHS), while others choose to remember the phrase Please Excuse My Dear Aunt Sally.

However, you choose to remember the PEMDAS rule is not as important as remembering the previously mentioned sub-rules? Why are the sub-rules to the PEMDAS rule so important? Because the sub-rules often make the difference between getting a correct or incorrect answer to a math problem.

The PEMDAS rule may not be perfect, but if you can remember the sub-rules, it can be a useful tool for helping you to correctly apply the math order of operations and getting correct answers on both simple and complex math problems provided that you know the important sub-rules.

Important Sub-Rules to the PEMDAS Rule:

1.) P: Perform operations inside of parenthesis or groups before you do anything else (if there are no groups or parentheses, you can skip this step).

2.) E: Next, after performing operations inside of parenthesis and groupings (if there are any), apply any exponents (if there are no exponents, you can skip this step).

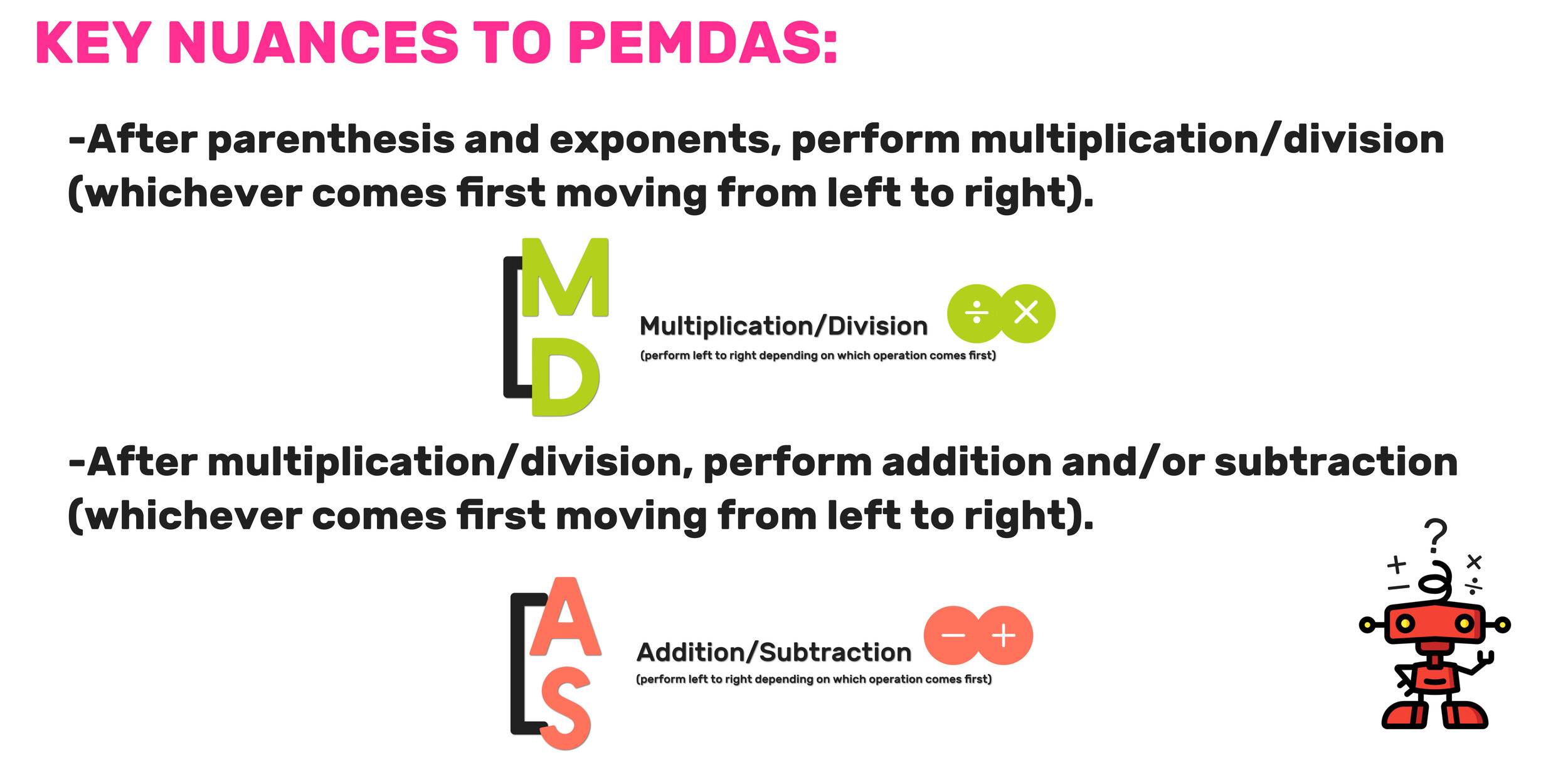
3.) M/D: Next, after the parentheses and groups and the exponents, perform multiplying/dividing from left to right based on whichever operation is first).

★ Just because M comes before D in the PEMDAS rule doesn’t mean that you will always perform multiplication before division.

4.) A/S: Finally, after multiplying and/or dividing, perform adding/subtracting from left to right based on whichever operation is first).

★ Just because A comes before S in the PEMDAS rule doesn’t mean that you will always perform addition before subtraction

★ = Extremely Important

PEMDAS Rule Examples

Now that you know what the PEMDAS rule for remembering the math order of operations stands for, it’s time to learn how to use the rule to solve math problems and get correct answers.

Why? Because there is zero educational value in remembering what the PEMDAS rule stands for if you have no clue how to apply it to math order of operations.

Remember that the PEMDAS rule is only useful if you also remember the key sub-rules that were displayed in the last section.

With the sub-rules in mind, the next section will work through several examples of how to correctly apply the PEMDAS rule when it comes to math order of operations and problem-solving.

PEMDAS Rule Ex. 1: (3+1) x 4

First, solve whatever is in the groupings (parentheses):

3+1=4

Next, multiply: 4 x 4 = 16

Final Answer: 16

PEMDAS Rule Ex. 2: 27 ÷ (8-5)^2

Again, perform operation inside of the groupings first.

Inside parentheses: 8-5 = 3.

The next step to evaluate the exponents: 3^2=9

The last step is to divide: 27÷9 = 3

Final Answer: 3

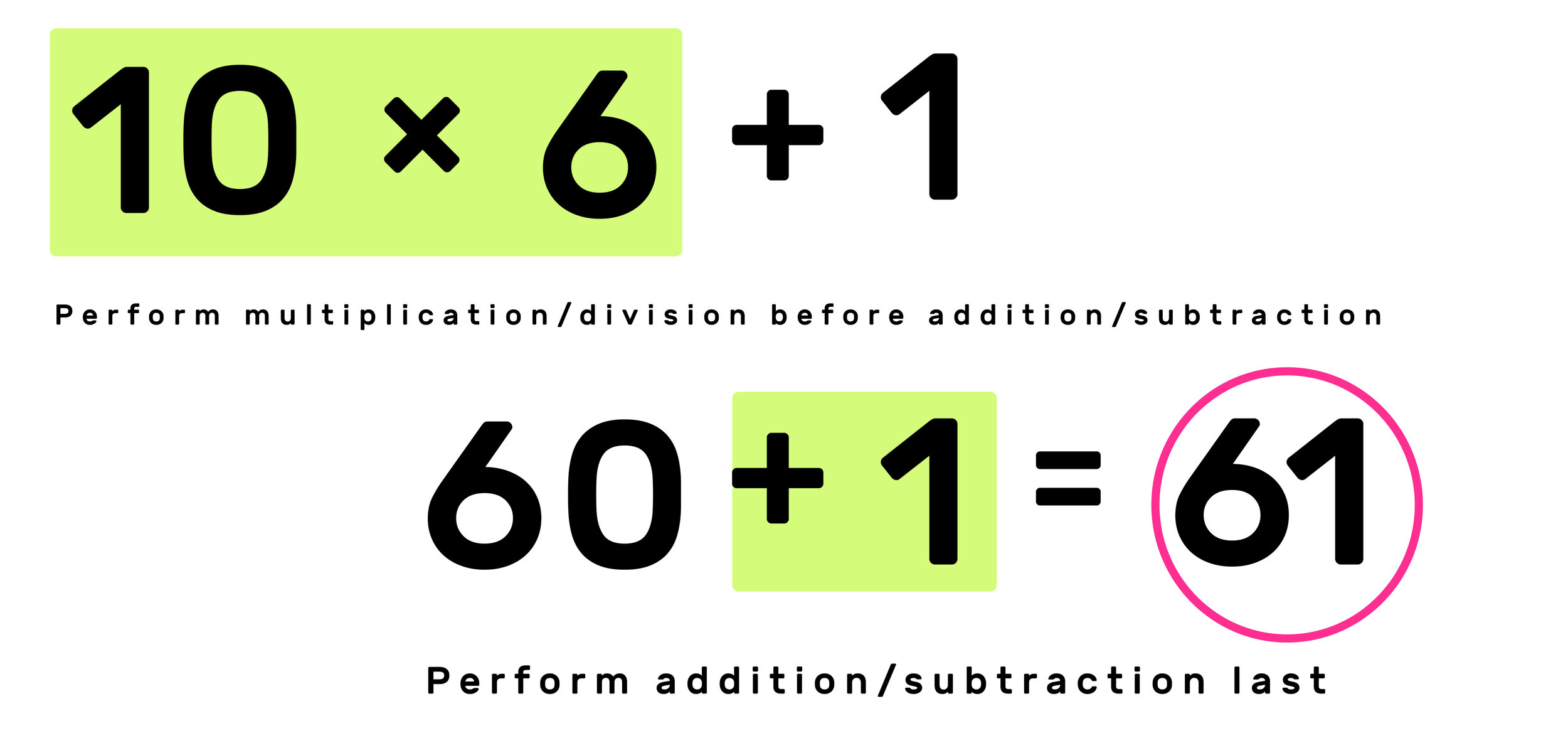
PPEMDAS Rule Ex. 3: 10 x 6 + 1

Notice that this example does not include groupings or any exponents. Therefore, you can skip the P and E in the PEMDAS rule and start with M/D.

Since multiplying/dividing comes before adding/subtracting, you can solve this problem by moving from left to right as follows:

10x6 = 60

60 + 1 = 61

Final Answer: 61

PEMDAS Rule Ex. 4: 75 - 10 x 5

According to the PEMDAS rule, multiplying/dividing comes before adding/subtracting so you can NOT solve this problem by moving from left to right.

The PEMDAS rule requires you to multiply first and then perform subtraction second as follows:

10 x 5 = 50

75 – 50 = 25

Final Answer: 25

PEMDAS Rule Ex. 5: 8 x 8 ÷ 16

Are you ready to apply the important sub-rules?

Notice that there are only two operations in this math example: multiplication and division.

Before we move forward, let’s revisit sub-rule #3:

3.) M/D: Next, after the parentheses and groups and the exponents, perform multiplying/dividing from left to right based on whichever operation is first).

★ Just because M comes before D in the PEMDAS rule doesn’t mean that you will always perform multiplication before division.

In this problem, you can solve by performing multiplication fist (the left-most operation) and then division second as follows:

8 x 8 = 64.

64 ÷ 16 = 4

Final Answer: 4

Note: If you followed the PEMDAS rule strictly and solved from left to right, you could still have ended up with 4 as the correct answer. However, this will not always be the case as we will in the next example.

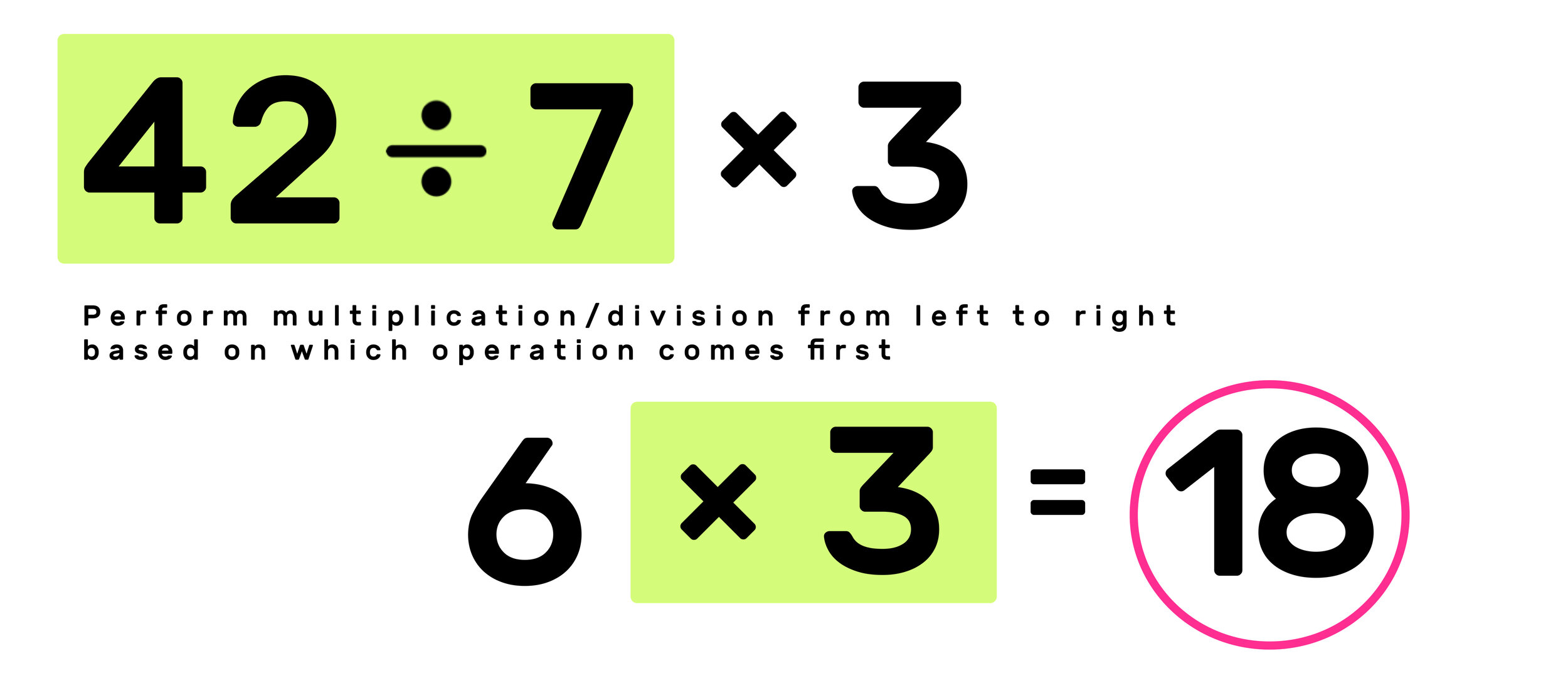
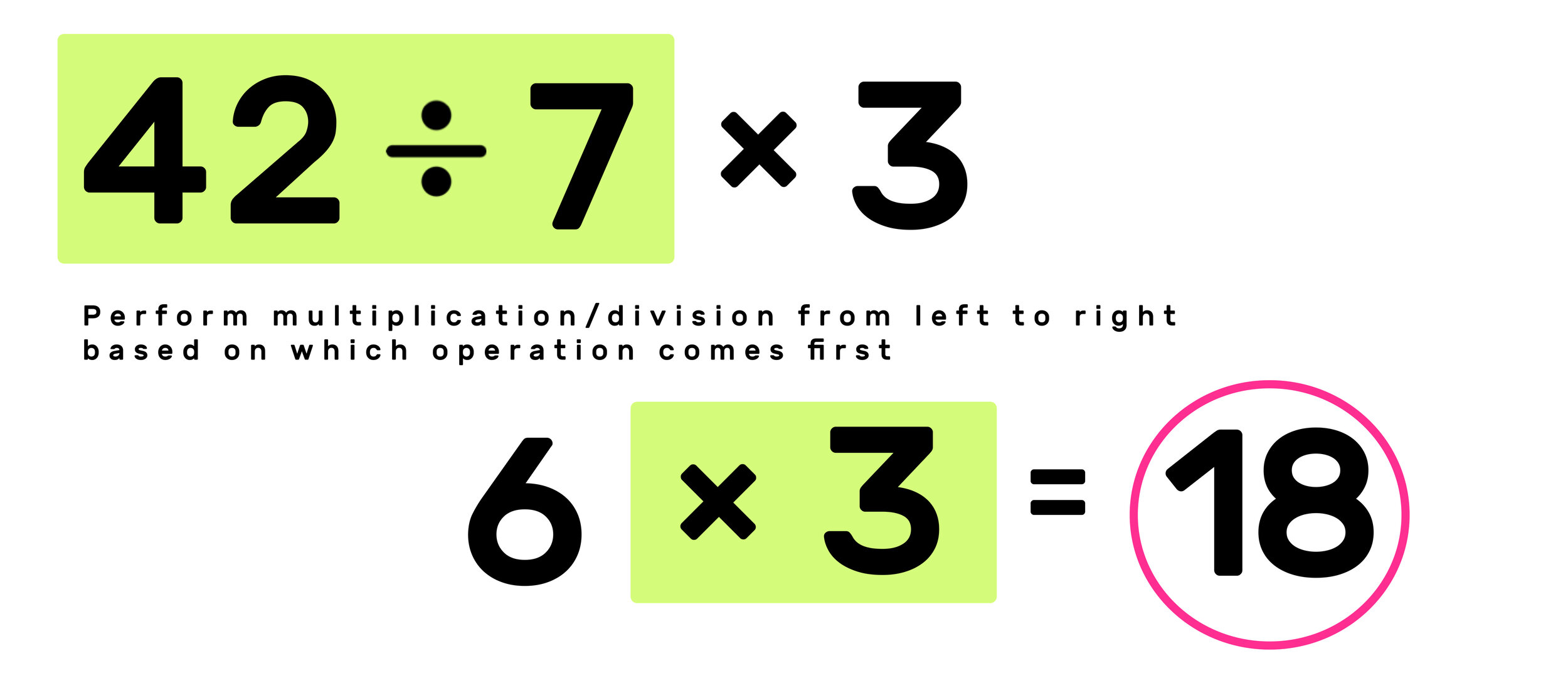
PEMDAS Rule Ex. 6: 42 ÷ 7 x 3

Let’s start by saying that many people will get this simple problem wrong because they forget the key sub-rules to the PEMDAS rule. They will make the mistake of strictly following the PEMDAS rule and performing multiplication before division (since M comes before D in PEMDAS).

Don’t make this mistake!

Remember that ★ Just because M comes before D in the PEMDAS rule doesn’t mean that you will always perform multiplication before division.

In this case, the only operations are multiplying and dividing. This time, division comes first, which is ok. You still solve the problem by moving from left to right as follows:

42÷7=6

6x3=18

Final Answer: 18

Why is 2 not the final answer? If you failed to apply the PEMDAS rule correctly, you may have made the mistake of performing multiplication before division as follows:

7 x 3 = 21

42 / 21 = 2 (THIS ANSWER IS WRONG!)

(Pro Tip: If this was a multiple-choice question, both 18 and 2 would both be choices. So, be careful!)

PEMDAS Rule: Why Is It Good to Know

It’s impossible to consistently solve math problems (both simple and complex) correctly without understanding how to apply the math order of operations and PEMDAS is an effective tool for recalling them in the right sequence—provided that you also remember the important sub-rules described above.

The PEMDAS rule and the math order of operations have gained tons of attention in recent years because of viral social media posts sharing seemingly simple math problems that garner thousands of responses (and incorrect answers) due to the fact that many adults can remember “PEMDAS” but not how to apply the actual PEMDAS rule (and corresponding sub-rules).

Since many people can’t figure out the right answer to these simple problems, they are inclined to leave comments and tag friends, which only makes the most more popular on social media.

PEMDAS Rule for Math Order of Operations: Conclusion

The PEMDAS rule is a popular memory tool for recalling the math order of operations. The rule stands for P: Parenthesis, E: Exponents, M: Multiplying, D: Dividing, A: Adding, S=Subtracting.

In general, operations are performed from left to right, but there are very important key sub-rules, namely (1) perform multiplying/dividing from left to right based on whichever operation is first), and perform adding/subtracting from left to right based on whichever operation is first).

Without understanding these sub-rules, the PEMDAS rule becomes extremely unreliable and can lead you to get the wrong answers to simple math problems (see PEMDAS Rule Ex. 6 above).

PEMDAS may not be the best way to remember how to correctly apply the math order of operations, but it can be a reliable tool if and only if you also remember the key sub-rules as well.