Calculating Percent Increase in 3 Easy Steps

Being able to calculate percent increase is an incredibly significant and useful math skill that can be applied in the classroom, on exams, and—most importantly—in the real world. While many students often perceive calculating percent increase as a difficult skill to master, it can actually be extremely easy.

(Looking for a Percent Increase Calculator to make a super fast calculation: Click here to access our free Percent Increase Calculator)

The following free Calculating Percent Increase step-by-step lesson guide will teach you how to calculate percent increase using a simple and effective three-step process. As long as you can remember the three steps and learn to apply them, you will be able to quickly and accurately calculate percent to correctly solve math problems.

Before you learn about calculating percent increase using our three-step process, let’s do a quick recap of some key vocabulary terms and definitions related to percents.

Looking to learn how to calculate percent decrease or percent change? Use the links below to download our free step-by-step guides:

Percent Definition

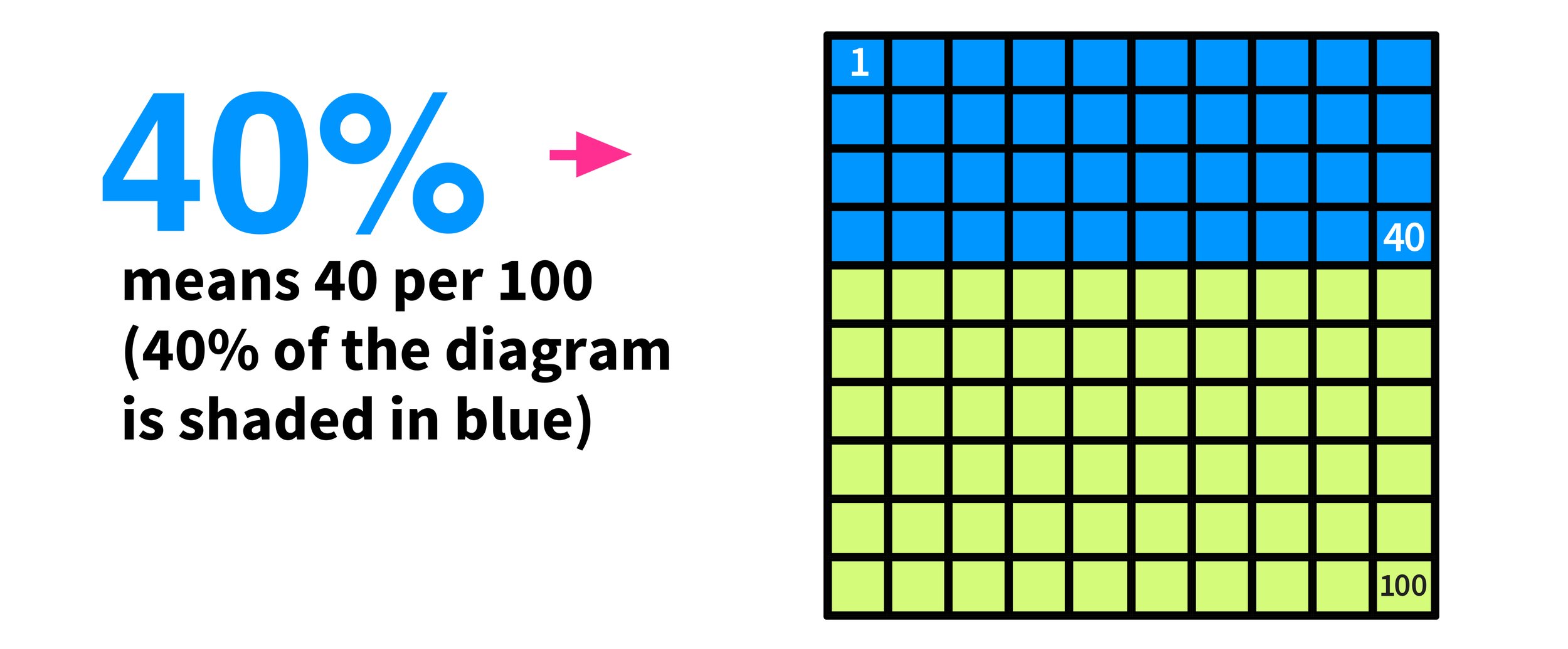
In math, a percent refers to parts per one hundred and the mathematical symbol for percent is %.

For example, 40% means 40 per 100. In the diagram below, 40% of the box is shaded in blue.

In other words, percent is a ratio of some value out of one hundred.

For example, 20% means 20 out of every 100. With this definition in mind, if 20% of 200 students have a test tomorrow, then 40 total students have a test tomorrow.

Percent Increase Definition

Now that you understand percent, what does percent increase mean?

In math, the percent increase between two numbers is the difference between the final number and the starting number. Percent increase is always expressed as a percentage of the first number.

Keep in mind that percent increase will always be represented as a percentage and will include a % symbol.

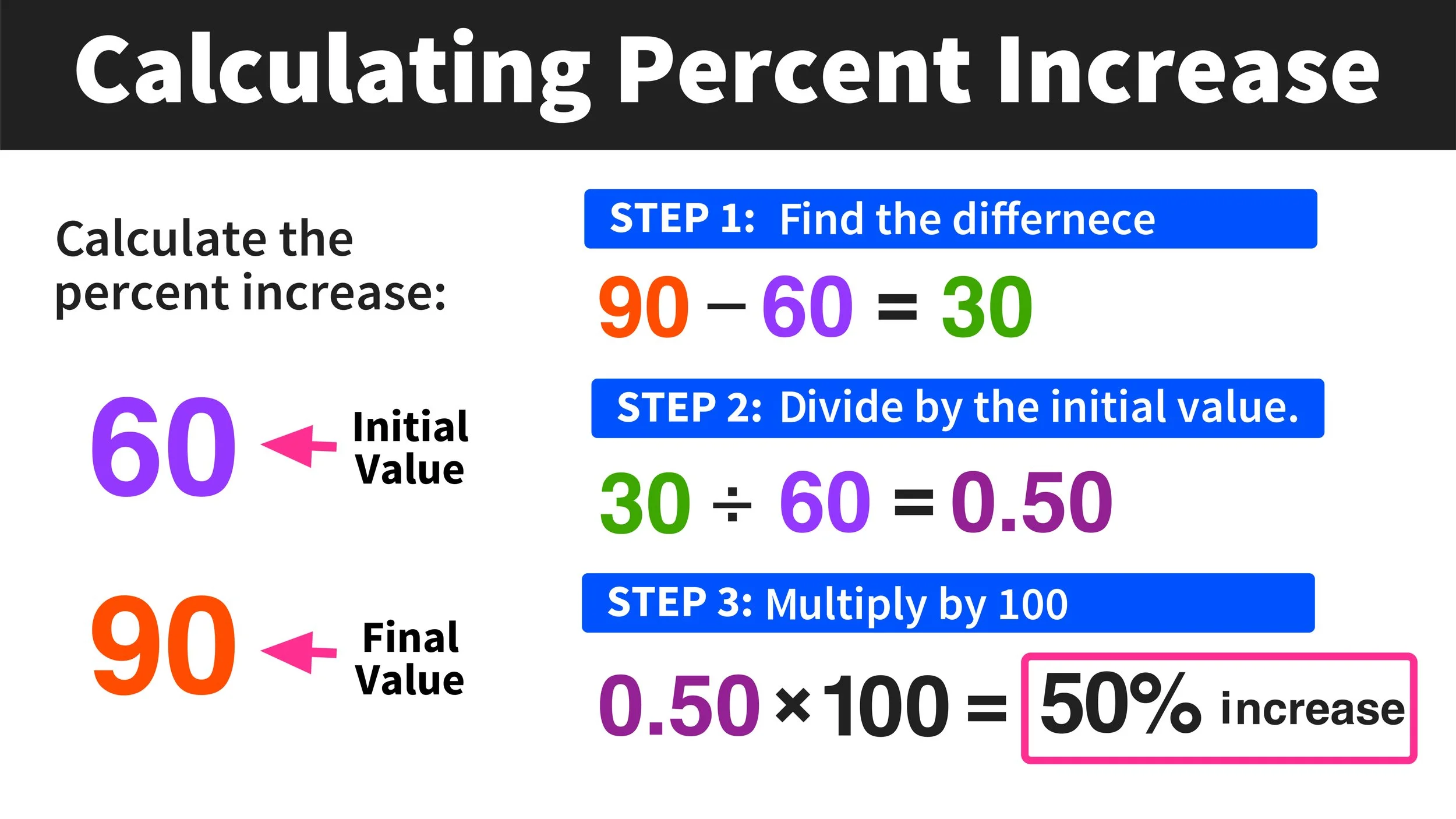
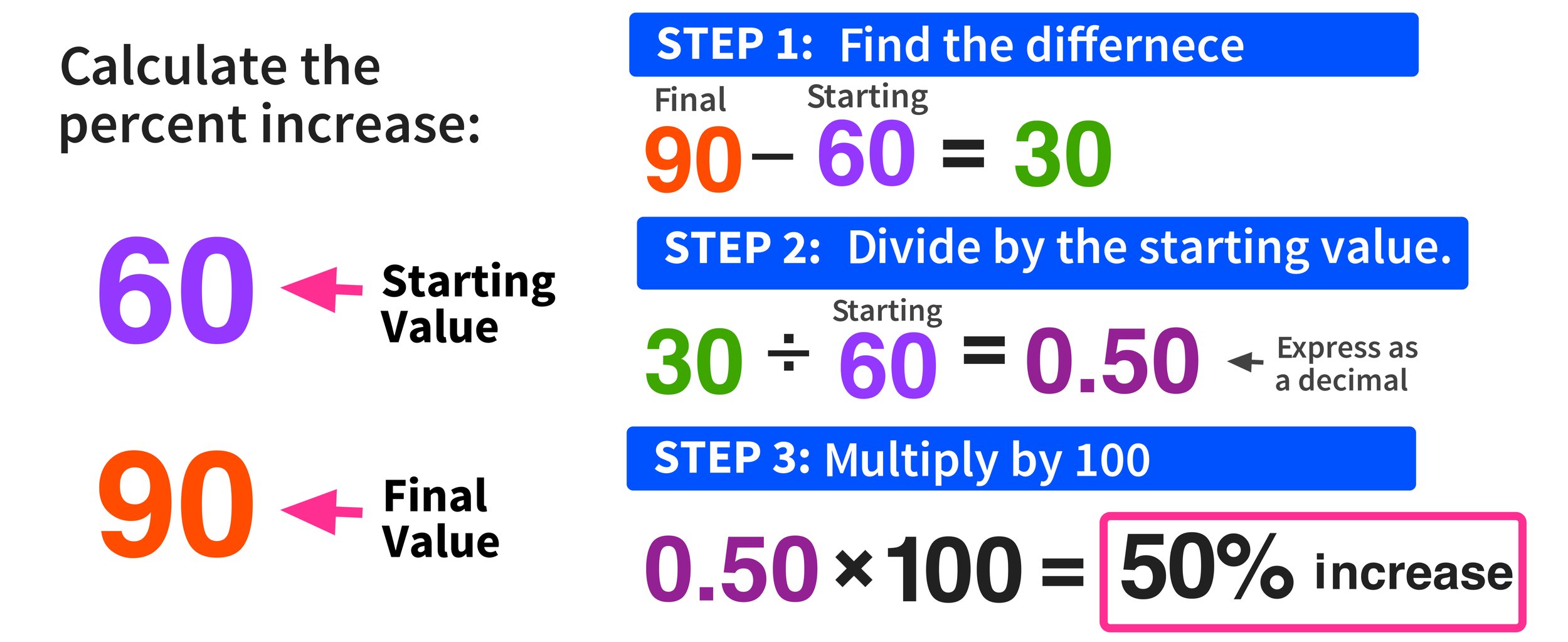
For example, if you had $60 dollars at the start of the week and $90 at the end of the week and wanted to find the percent increase, the final number would be 90 and the starting number would be 60.

Identifying the starting number and the final number is relatively simple and it is key for solving percent increase problems.

Calculating Percent Increase

Now you are ready to learn to calculate percent increase using our easy three-step process.

Let’s take a close look at the previous scenario:

Calculating Percent Increase Example #1

For the first example, let’s find the percent increase for the following scenario:

If your total savings of $60 at the start of the week has grown to $90 by the end of the week, what is the percent increase?

Here is where our three-step process comes in:

Step 1: Find the difference of the values by subtracting the starting value from the final value.

In this case, the final value minus the starting value can be calculated as follows:

90 – 60 = 30

So, the difference of the two values would be 30 in this example. Note that, when calculating percent increase, you will always be subtracting the smaller value from the larger value.

Step 2: Divide the difference by the starting number.

The next step is to take the difference (30 in this example) and divide it by the starting number (60 in this example) as follows:

30/60 = 0.50

Always express your answer as a decimal (doing this will make your life much easier when you get to step three).

Step 3: Multiply by 100

The final step is to multiply the decimal result from step two by one hundred and express the final result as a percent.

0.50 x 100 = 50

Final Answer: 50% Increase

That’s all there is to it! By using the three steps, you can conclude that there was a 50% increase in how much money you had from the start of the week to the end of the week.

Confused? That’s totally fine. Let’s take a look at another example where we will calculate percent increase using the three-step process.

Looking for a free Percent Increase Calculator?

If you need a faster way to calculate the percent increase between two numbers, check out our free Percent Increase Calculator tool, which lets you input the starting and final values to get an instant answer!

▶ Click here to access our free Percent Increase Calculator for students

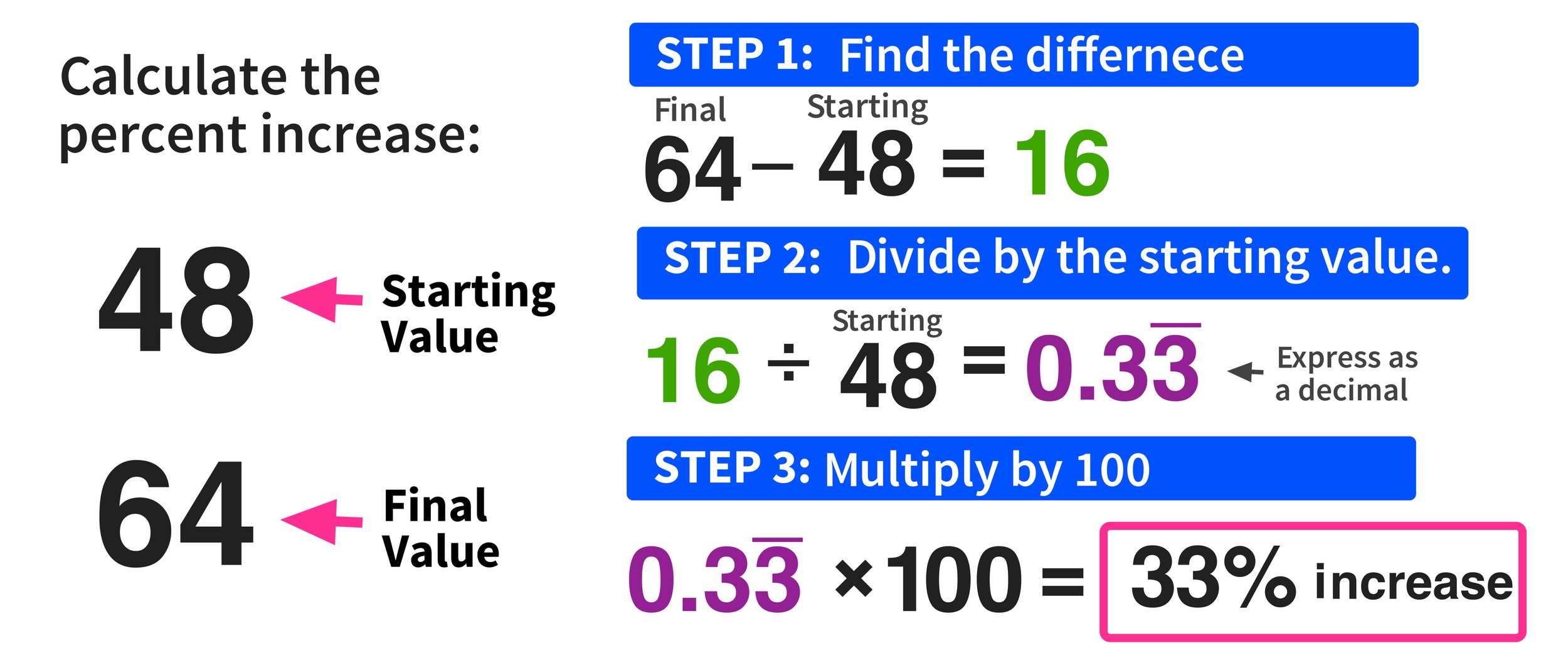
Calculating Percent Increase Example #2

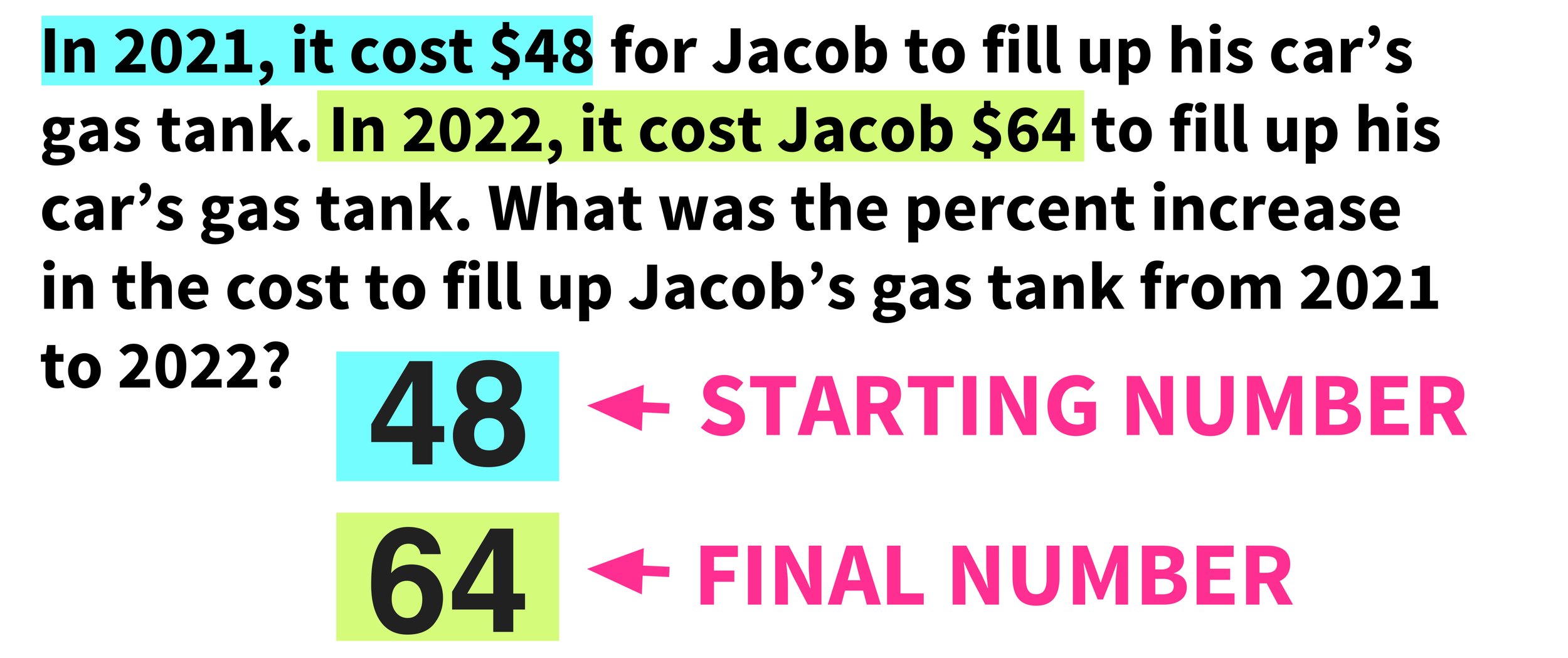
In 2021, it cost $48 for Jacob to fill up his car’s gas tank. In 2022, it cost Jacob $64 to fill up his car’s gas tank. What was the percent increase in the cost fill up Jacob’s gas tank from 2021 to 2022?

To solve this problem, note that the starting value is 48 and the final value is 64.

Step 1: Find the difference of the values by subtracting the starting value from the final value.

In this example, the final value minus the starting value can be calculated as follows:

64– 48 = 16

Step 2: Divide the difference by the starting number.

For step two, take the difference (16 in this example) and divide it by the starting number (48 in this example) as follows:

16/48 = 0.3333333

Notice that the result is a repeating decimal, which is ok. You can round the result to the nearest hundredths decimal place to make things easier. In this case, you can round the result to 0.33

Step 3: Multiply by 100

The last step is to multiply the decimal result from step two by one hundred and express the final result as a percent. So…

0.33 x 100 = 33

Final Answer: 33% Increase

We’re all finished! We have concluded that there was a 33% increase in the cost of filling Jacob’s gas tank from 2021 to 2022.

Hopefully, you are feeling better about calculating percent increase using the three-step method. But, if you could still use a little more practice, let’s take a look at one last example.

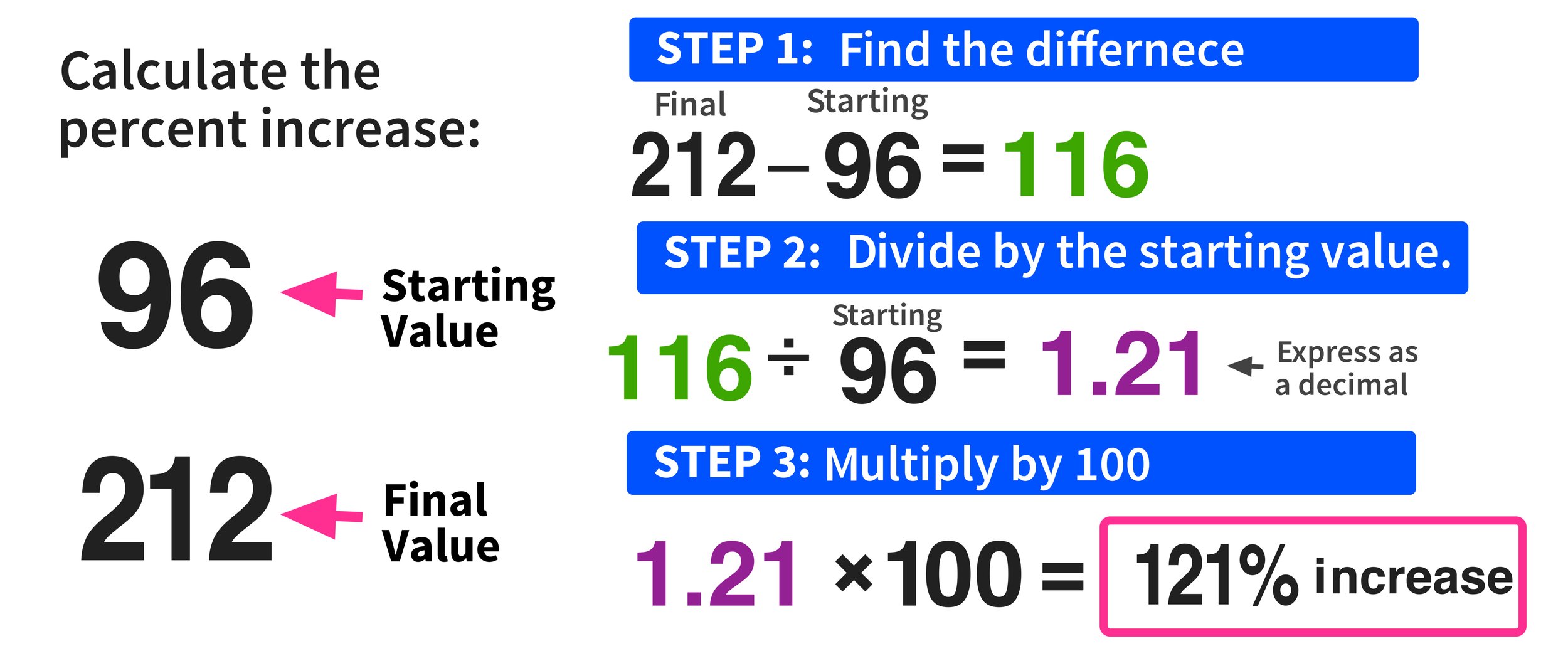
Calculating Percent Increase Example #3

Last school year, 96 students tried out for the Varsity Baseball Team at Delta High School. This year, 212 students tried out. What was the percent increase in students who tried out for the Varsity Baseball Team?

Just like the last two examples, you can solve this problem by following the three-step process:

Step 1: Find the difference of the values by subtracting the starting value from the final value.

In this example, the final value minus the starting value can be calculated as follows:

212– 96 = 116

Step 2: Divide the difference by the starting number.

Did you notice that the result from step one is actually larger than the starting value? This occurrence is totally fine and will not prevent the three-step process from working. So, let’s continue with step two as follows:

Find the difference (116 in this example) and divide it by the starting number (96 in this example) as follows:

116/96 = 1.2083333

Just like in example 2, you can round the result to the nearest hundredths decimal place to make things easier. In this case, you can round the result to 1.21

Step 3: Multiply by 100

The last step is to multiply the decimal result from step two by 100 and express the final result as a percent. So…

1.21 x 100 = 121

Final Answer: 121% Increase

All done! Notice how, in this example, the percent increase is over 100%, which just means the ending value was more than double the starting number.

By now, you should be feeling confident in your ability to calculate percent increase using three-step process. However, if you would like some more practice, I recommend that you work through examples one through three again on your own.

Conclusion: Calculating Percent Increase

You can calculate percent increase given any two values by using the following 3-step method:

Step 1: Find the difference of the values by subtracting the starting value from the final value.

Step 2: Divide the difference by the starting number. Express result as a decimal.

Step 3: Multiply by 100. Express result as a %.

What about Calculating Percent Decrease and Percent Change?

Learn how to calculate a percent decrease or a percent change between two numbers using our free step-by-step guides. Click the links below to get started.