How to Divide Decimals Explained in 3 Easy Steps

Step-by-Step Guide: How to Divide Decimals by Whole Numbers and How to Solve Decimal Divided by Decimal Problems

In math, it is important to be able to work with and perform operations on decimals, which are numbers in the base-10 system that include a point that separates the whole number(s) from the attached fractional parts. For example, the number 2.5 is a decimal number that represents two and a half.

One of the more challenging operations to perform with decimals is division. However, if you know how to divide whole numbers, then you can easily learn how to divide decimals using just a few simple steps. Note that there are two different cases when it comes to dividing decimals: a decimal divided by a whole number and a decimal divided by another decimal. We will cover both cases in this guide.

Below are quick links to each section of this free Step-by-Step Guide on How to Divide Decimals:

• Quick Review: What is a Dividend? What is a Divisor?

• Quick Review: Long Division

• How to: Divide Decimals by Whole Numbers

• How to: Dividing Decimals by Decimals

• Extra Practice: Free Dividing Decimals Worksheet

While learning how to divide with decimals can be intimidating at first, it is a math skill that you can easily learn with practice following a simple 3-step process. This free dividing with decimals tutorial will teach you everything you need to know about how to divide with decimals, including several step-by-step practice problems for both dividing decimals by whole numbers and dividing decimals by decimals.

But, before we dive into our practice problems, let’s do a quick recap of some important vocabulary terms related to division as well as a quick review of how to perform long division. If you are already comfortable with the review information, you can use the quick links above to skip ahead to the section that best meets your needs.

What is a dividend? What is a divisor?

In this guide on dividing decimals, we will be using the terms dividend and divisor often, so make sure that you are familiar with what they mean:

• When dividing two numbers, the dividend is the number that is being divided.

• When dividing two numbers, the divisor is the number of parts the dividend is being divided into.

For example, consider the division problem: 248 ÷ 8

• 248 is the dividend because it is the number being divided

• 8 is the divisor because 248 is being divided into 8 parts.

This example is illustrated in Figure 01 above.

Because this guide will be teaching you how to divide decimals without using a calculator, we will be using long division to solve problems. Therefore, it is important that you are familiar with the divisor/dividend notation shown in Figure 01 above, where: 248 ÷ 8 → 8 | 248

Now that you know how to identify a dividend and a divisor and the divisor/dividend notation, lets do a quick review of how to perform long division using the same example of 248 ÷ 8.

Figure 02 above shows a step-by-step review of how to use long division to determine that 248 ÷ 8 = 31.

If you are not comfortable with performing long division, then we recommend that you pause now and do a deeper review before moving forward with this tutorial on how to divide decimals.

How to Divide Decimals by Whole Numbers

The first set of examples in this dividing decimals tutorial will focus on how to divide decimals by whole numbers and will include examples for when the dividend is the whole number and when the divisor is the whole number as well.

How to Divide Decimals by Whole Numbers

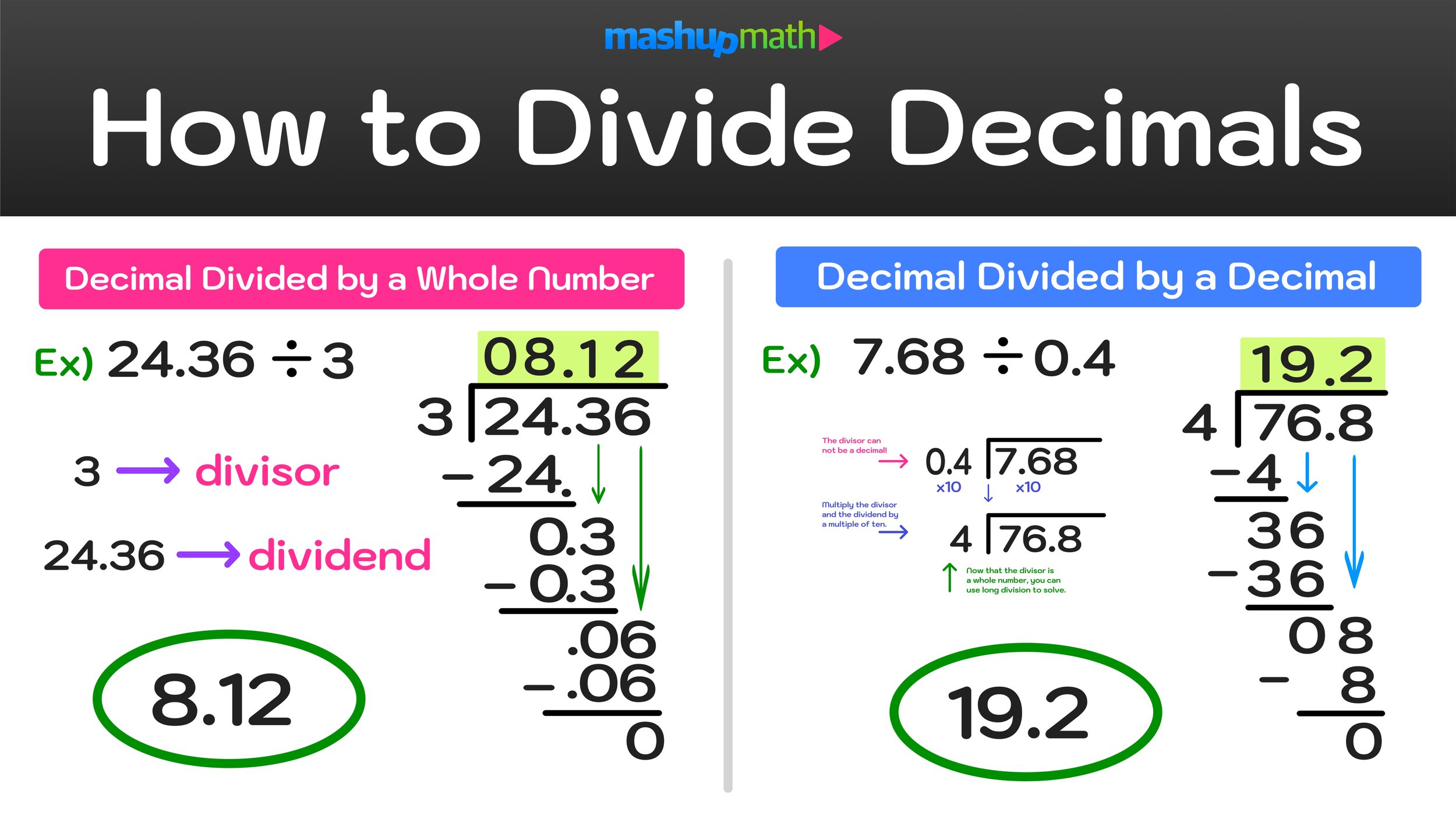
Example #1: 1.5 ÷ 2

Let’s start off with a simple example that you could probably solve without the use of long division (although we will solve it using long division anyway so that you can start to become more familiar with our 3-step process for dividing decimals).

For this example, and all of the examples that follow, you will be using the following three step method for dividing decimals:

• Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

• Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

• Step Three: Use long division to solve.

We will be applying this 3-step process of all of the dividing decimals practice problems in this guide, so don’t get intimidated if you are a little confused right now. The process will make more sense and be easier to apply after we work through a few examples.

Lets start with the first step:

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

In the case of 1.5 ÷ 2

• 2 is the divisor

• 1.5 is the dividend

As shown in Figure 03 above, it is clear that the divisor is 2, which is indeed a whole number, so, for this example, we can skip the second step and move right onto Step Three.

Also notice that in Figure 03 above, we rewrote 1.5 as 1.50 (they both mean the same thing). Adding extra zeros after the last digit of a decimal does not change the number and often helps you to perform long division, as you will see in the next step.

Step Three: Use long division to solve.

All that you have to do now is use long division to solve the problem. You can click play on the video below to see an animated step-by-step breakdown of how to perform the long division for this problem.

Based on the video and the illustrated summary shown in Figure 04 below, you can see that:

Solution: 1.50 ÷ 2 = 0.75

This solution should make sense because dividing 1.50 in half will result in 0.75. Before moving onto another similar example of a decimal divided by a whole number, we encourage you to review the above review as we will not include videos for every example.

Dividing Decimals by Whole Numbers

Example #2: 24.36 ÷ 3

For this next example, we will be using the exact same three-step approach as Example #1.

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

For this example:

• 3 is the divisor

• 24.36 is the dividend

Since the divisor in this example is a whole number (3), we can skip the second step just like we did in the previous example and move onto the third and final step.

Step Three: Use long division to solve.

To solve the second example, perform long division just as you did to solve Example #1. Remember to follow your steps carefully and to line up your decimal points.

The entire process of using long division to solve 24.36 ÷ 3 is illustrated in Figure 05 below.

After completing Step Three, we can conclude that:

Solution: 24.36 ÷ 3 = 8.12

Now, lets look at a few examples of a decimal divided by a whole number where the divisor is not a whole number.

How to Divide Decimals by Whole Numbers

Example #3: 92 ÷ 2.3

For this third example of dividing decimals by whole numbers, we will again be using the same three-step method as the previous two examples (as well as all on the examples that will follow this one), except that this time we will not be able to skip the second step.

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

In this case:

• 2.3 is the divisor

• 92 is the dividend

Since the divisor in this example is 2.3, which is not a whole number, we will have to move onto the second step (which we were able to skip in the previous two examples).

Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

When it comes to dividing decimals, we cannot have a decimal as a divisor. However, we can multiply both the divisor and the dividend by the same multiple of ten to transform the divisor into a whole number and still have a proportional relationship.

Since the final digit of 2.3 is in the tenths place value slot, we will multiply both the divisor (2.3) and the dividend (92) by 10 as shown below and in Figure 06:

• 2.3 x 10 = 23

• 92 x 10 = 920

*Remember that what you do to one number, you must do to the other number. If you forget to multiply both the dividend and the divisor by 10, you will get the wrong answer.

Step Three: Use long division to solve.

After completing Step Two, all we have to do is use long division to solve 920 ÷ 23.

The step-by-step process for using long division to divide 920 by 23 is shown in Figure 07 below.

Finally, we can say that:

Solution: 92 ÷ 2.3 = 40

Next, lets look at one final example of how to divide decimals by whole numbers before we move onto learn all about dividing decimals by decimals.

How to Divide Decimals by Whole Numbers

Example #4: 16 ÷ 6.25

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

For the fourth example, the divisor is a decimal and the dividend is a whole number.

• 6.25 is the divisor

• 16 is the dividend

Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

Since the divisor is a decimal (6.25), we will have to multiply both the divisor and the dividend by the same multiple of ten.

And since, in this example, the final digit of the divisor, 6.25, is in the hundredths place value slot, we will multiply both the divisor and the dividend by 100 as shown below and in Figure 08.

• 6.25 x 100 = 625

• 16 x 100 = 1,600

After completing long division, we can conclude that:

Solution: 16 ÷ 6.25 = 2.56

Now we will move on from dividing decimals by whole numbers to learning how to divide decimals by decimals.

Dividing Decimals by Decimals

This section of our guide focused on dividing decimals by decimals. If you used the quick links at the top of the page to skip to this section, we recommend working through the examples in the dividing decimals by whole numbers section above, because it will help you to better understand how to use the following three-step method for dividing decimals by decimals:

• Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

• Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

• Step Three: Use long division to solve.

Just as the previous section on dividing decimals by whole numbers, we will be following the same steps for dividing decimals by decimals.

Lets go ahead and dive into the first example.

How to Divide with Decimals

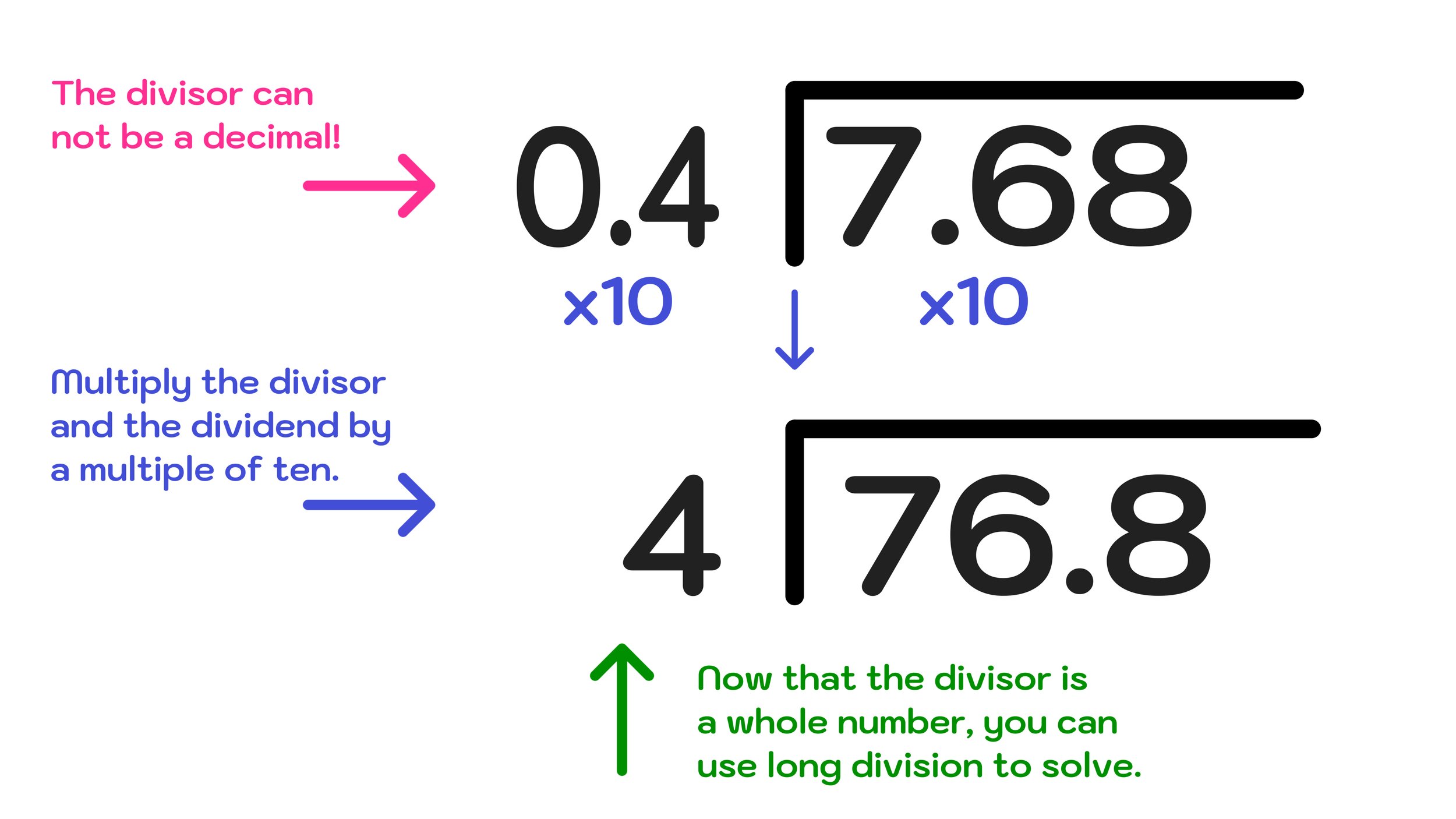
Example #1: 7.68 ÷ 0.4

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

For this first example:

• 0.4 is the divisor

• 7.68 is the dividend

For all of the examples in this section, we will be dividing decimals by decimals, so it will always be the case that the divisor is not a whole number. Therefore, you will always have to move onto Step Two, where you will use multiplication to transform the divisor into a whole number.

Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

Again, a decimal divided by a decimal can not be solve using long division when the divisor is not a whole number. Luckily, you can easily transform the divisor into a whole number by multiplying both the divisor and the dividend by a multiple of ten and still have a proportional relationship where you can use long division to solve the problem.

Since the final digit of 0.4 is in the tenths place value slot, you can multiply both the divisor (0.4) and the dividend (7.68) by 10 as shown below and as illustrated in Figure 09.

• 0.4 x 10 = 4

• 7.68 x 10 = 76.8

*Always remember that whenever you multiply the divisor by a multiple of 10, you also have to multiply the dividend by that same multiple of 10. If you forget to multiply both by the same multiple of 10, you will not be able to correctly solve the problem.

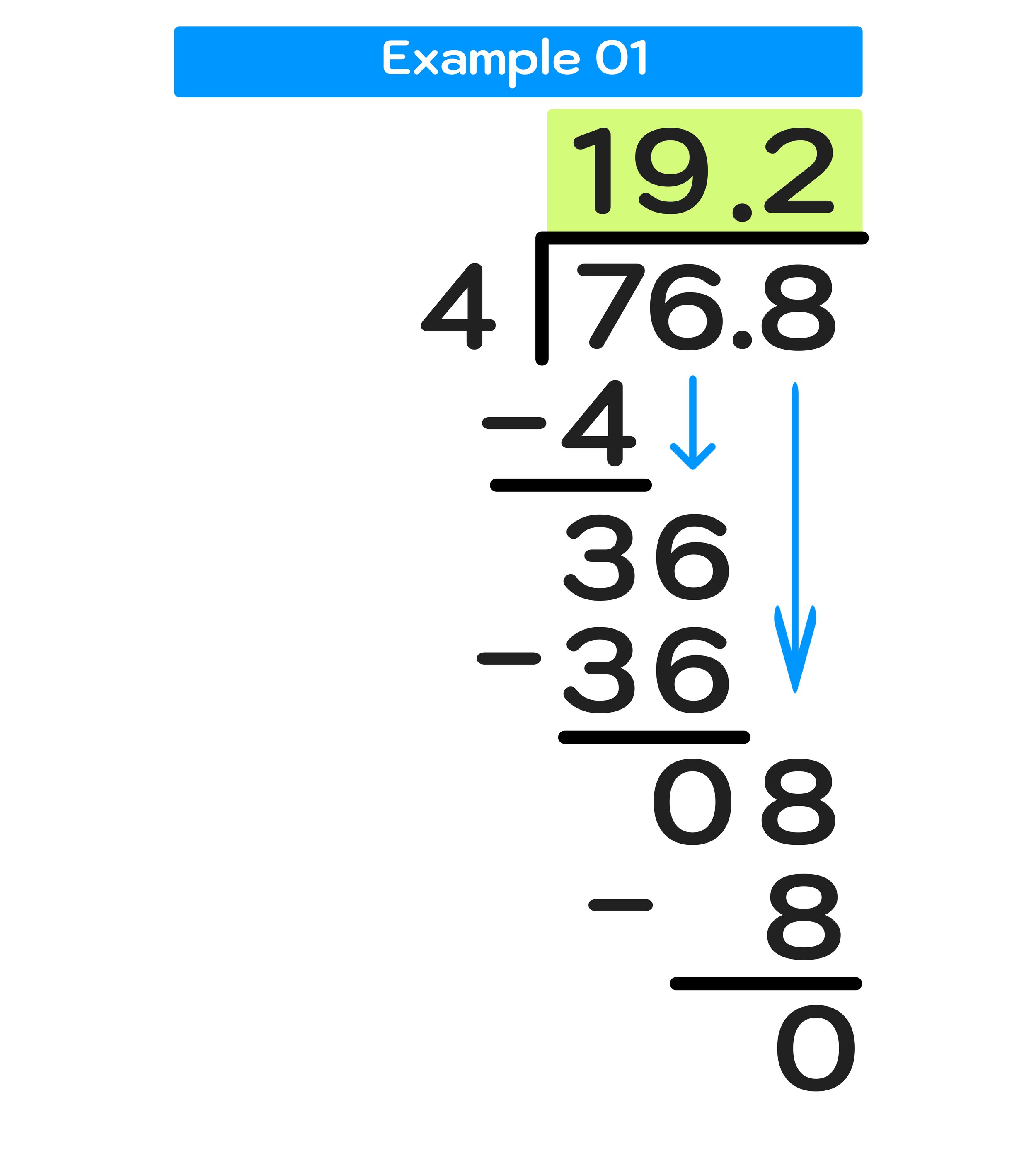
Step Three: Use long division to solve.

Now that you have transformed the divisor into a whole number, you can use long division to solve the problem. You can click play on the video below to see an animated step-by-step breakdown of how to perform the long division for this problem.

Based on the video and the illustrated summary shown in Figure 10 below, we can conclude that:

Solution: 7.68 ÷ 0.4 = 19.2

Before you continue onto the next example of how to divide decimals by decimals, we highly recommend that you review the step-by-step long division tutorial above as we will not include video tutorials for every problem.

How to Divide Decimals by Decimals

Example #2: 38.4 ÷ 0.24

Just like the previous example, we will use our three step method to solve a decimal divided by a decimal problem.

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

For this first example:

• 0.24 is the divisor

• 38.4 is the dividend

Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

Since the divisor, 0.24, is a decimal, you will have to multiply it (and the dividend) by a power of ten to make it a whole number. Since the last digit of 0.24 is in the hundredths place value slot, we will multiply both the divisor and the dividend by 100 as shown below and in Figure 11.

• 0.24 x 100 = 24

• 38.4 x 100 = 3,840

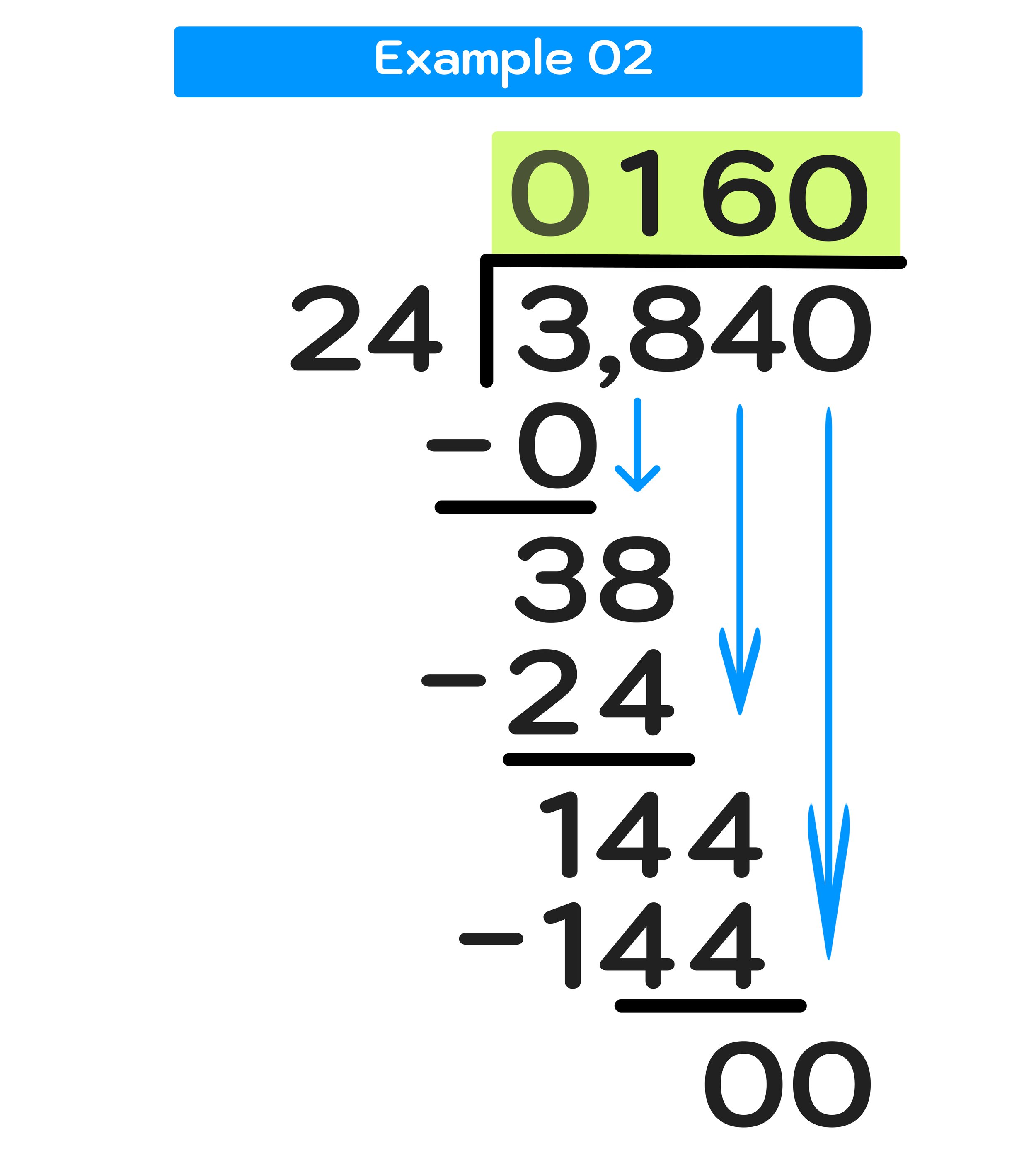
Step Three: Use long division to solve.

Finally, you now have a divisor that is a whole number, so you can simply use long division to solve 3,840 ÷ 24 to find the solution to this problem, as illustrated in Figure 12 below.

Solution: 38.4 ÷ 0.24 = 160

Now, lets work through one final example.

How to Divide Decimals by Decimals

Example #3: 4.76 ÷ 1.36

Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

For this first example:

• 1.36 is the divisor

• 4.76 is the dividend

Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

Since the divisor, 1.36, is a decimal, you will have to multiply it (and the dividend) by 100 to transform it into a whole number (we chose to multiply the dividend and the divisor by 100 because the last digit of 1.36 is in the hundredths decimal slot).

• 1.36 x 100 = 136

• 4.76 x 100 = 476

Step Three: Use long division to solve.

Now you can find the solution by using long division to solve 476 ÷ 136 as shown in Figure 13 below.

Solution: 4.76 ÷ 1.36 = 3.5

Dividing Decimals Worksheet

Are you looking for some extra practice with solving problems involving dividing decimals?

You can click the link below to download your free Dividing Decimals Worksheet, which includes a complete answer key so you can check your work. Be sure to apply the three-step process shared in this guide (and also featured on the worksheet) when solving the problems.

▶ Download Your Free Dividing Decimals Worksheet (w/ Answer Key)

▶ Access More Free Topic-Specific Math Worksheets

Conclusion: How to Divide Decimals

Learning how to divide decimals by whole numbers or other decimals is an important math skill that every student will eventually have to learn how to do.

While dividing decimals can seem challenging, as long as you know how to perform long division, you can easily solve dividing decimals problems by using the following 3-step approach:

• Step One: Identify the dividend and the divisor and determine whether or not the divisor is a whole number (if it is, move onto Step Three).

• Step Two: If the divisor is not a whole number, multiply it by a multiple of 10 to make it a whole number (multiply tenths by 10, hundredths by 100, thousandths by 1,000, etc.). Whatever multiple of 10 that you multiplied the divisor by, you must also multiply the dividend by.

• Step Three: Use long division to solve.

By working through the examples in this guide as well as the practice problems on the free dividing decimals worksheet, you will gain invaluable practice and experience with dividing decimals, which will make solving problems where you have to divide decimals a simple and easy task.

Keep Learning:

How to Convert a Decimal to a Fraction in 3 Easy Steps (Free Step-by-Step Guide for Students)