How to Simplify Radicals in 3 Easy Steps
Math Skills: How to Simplify a Radical Using a 3-Step Strategy
Every math student must learn how to work with numbers inside of a radical (√) at some point. While working with perfect squares inside of radicals can be quite easy, the task becomes a bit trickier when non-perfect squares are involved and you have to simplify.
While learning how to simplify a radical of a non-perfect square may seem challenging, it’s actually a relatively easy math skill to learn as long as you have a strong understanding of perfect squares and factoring (we will review both of these topics in this guide).
This free How to Simplify Radicals Step-by-Step Guide will teach you how to simplify a radical when the number inside of it is not a perfect square using a simple 3-step strategy that you can use to solve any problem involving simplifying radicals.
The following topics and examples will be covered in this guide:
You can use the quick-links above to jump to any section of this guide. However, we highly recommend that you work through each section in order, including the following review section where we will quickly recap some important features of perfect squares, non-perfect squares, factors, and the properties of radicals. This review will recap some important vocabulary terms and prerequisite math skills that you will need to be successful with this new math skill.
But, before you learn how to add fractions, let’s do a quick review of some key characteristics and vocabulary terms related to fractions before we move onto a few step-by-step examples of how to add fractions.
Let’s get started!
Radicals, Perfect Squares, and Non-Perfect Squares
Before you learn how to simplify a radical, it’s important that you understand what a radical is and what the difference between a perfect square and a non-perfect square is.
Definition: In math, a radical (√) is a symbol that is associated with the operation of finding the square root of a number.
Finding the square root of a number means finding an answer to the question: “What number times itself results in the original number?” For example, the square root of 25 (or √25) is equal to 5 because 5 times 5 equals 25.
Definition: In math, a perfect square is a number that is the square of any integer. This means that a perfect square is a number that can be represented as the product of an integer squared (or an integer multiplied by itself). For example, 36 is a perfect square because 6 times 6 equals 36.
Note that perfect squares are unique and there are not that many of them. In fact, most numbers are considered non-perfect squares because they can not be represented as an integer times itself.
Figure 01: What is a perfect square?
Definition: In math, a non-perfect square is a number that is not the square of any integer. This means that a non-perfect square is a number that can’t be represented as the product of an integer squared (or an integer multiplied by itself). For example, 20 is not a non-perfect square because it is impossible to take an integer and multiply by itself to get a result of 20.
Again, most numbers are non-perfect squares. When we see these types of numbers inside of a radical, they can be simplified or expressed as decimal numbers, but never as an integer.
The chart in Figure 02 below shows all of the perfect squares and non-perfect squares up to 144. Note that the numbers highlighted in orange are all perfect squares. For example:
49 is a perfect square (because 7 x 7 = 49)
8 is a non-perfect square
Figure 02: Before you learn how to simplify a radical, you must be familiar with the perfect squares.
For quick reference, here is a list of the perfect squares up to 144:
1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144
Now that you are familiar with radicals, perfect squares, and non-perfect squares, you are ready to learn how to simplify radicals.
How to Simplify Radicals in 3-Easy Steps
Now you are ready to learn how to simplify a radical using our easy 3-step radical.
Let’s start by considering a problem where you are asked to simplify c. You should notice that 16 is a perfect square, so you can easily conclude that √16 = 4 (because 4 x 4 =16).
This kind of problem is pretty easy when the number inside of the radical is a perfect square, but what happens when it is not a perfect square? For example, what if you were dealing with a non-perfect square like 12 inside of the radical? How can you simplify √12? This guide will teach you how to do just that (i.e. how to simplify a radical containing a non-perfect square).
To simplify radicals like √12, we will use the following 3-step strategy:
Step One: List all of the factors of the number inside of the radical
Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.
Step Three: Separate the original radical into two radicals and simplify.
Does this sound confusing? The process gets easier with practice. Let’s go ahead and apply these three steps to √12 as follows:
Step One: List all of the factors of the number inside of the radical
First, we will list all of the factors of 12:
Factors of 12: 1, 2, 3, 4, 6, 12
Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.
From the list of factors above, we can see that only 4 is a perfect square and it is the only perfect square that is a factor of 12. This is important because we will need to find at least one factor that is a perfect square in order to simplify a radical of a non-perfect square.
4 is a factor of 12 and a perfect square
4 x 3 = 12
Figure 03: How to split up a radical.
Step Three: Split the original radical into two radicals and simplify.
For the final step, what do we mean by “split up the radical”?
Figure 03 above illustrates the following property of radicals:
√(ab) = √a x √b (as long as a>0 and b>0)
This means that you can separate a radical into the radicals of two of its factors. In the case of √12, we can rewrite it as follows:
√12 = √(4 x 3) = √4 x √3
Now, notice that √4 is equal to 2 (because 4 is a perfect square), so we can rewrite the result as follows:
√12 = = √4 x √3 = 2 x √3
From here, we can not simplify this radical any further, so we can conclude that:
Final Answer: √12 = 2√3
Figure 04 below illustrates how we solved this problem using our 3-step strategy:
Figure 04: How to Simplify Radicals in 3 Easy Steps.
Now that you have learned the 3-step strategy for how to simplify radicals, let’s gain some experience using this strategy to solve a few practice problems.
How to Simplify Radicals Example #1
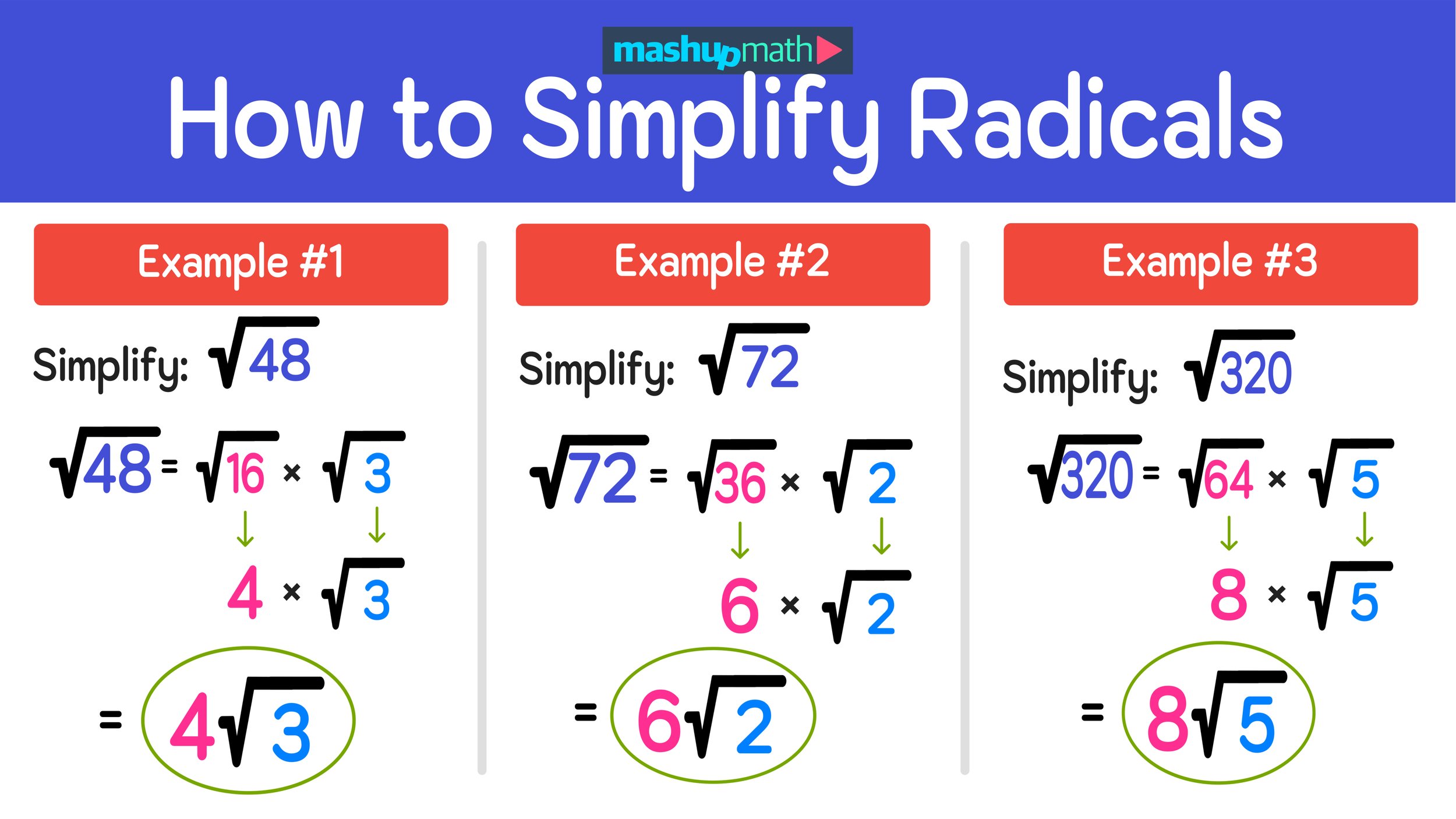
Example #1: Simplify √72
For this first example (and all of the examples in this guide) we will use our 3-step strategy to simplify the given radical as follows:
Step One: List all of the factors of the number inside of the radical
For the first step, let’s list all of the factors of 72:
Factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72
Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.
Next, take a look at the list of factors of 72. Notice that 72 has three facts that are perfect squares other than 1: 4, 9, and 36. In cases like this, you have to choose the largest perfect square, which is 36 for this example.
36 is a factor of 72 and 36 is a perfect square
36 x 2 = 72
Step Three: Split the original radical into two radicals and simplify.
Finally, we can split the radical into the radicals of two of its factors (namely 36 and 2) as follows:
√72 = √(36 x 2) = √36 x √2
Since 36 is a perfect square (36 = 6x6), we know that √36=6, so we can say that:
√72 = = √36 x √2 = 6 x √2
Now we can make the following conclusion:
Final Answer: √72 = 6√2
Figure 05 below illustrates how we simplified √72 for this first example.
Figure 05: How to Simplify Radicals Explained
Let’s continue onto another practice problem!
How to Simplify Radicals Example #2
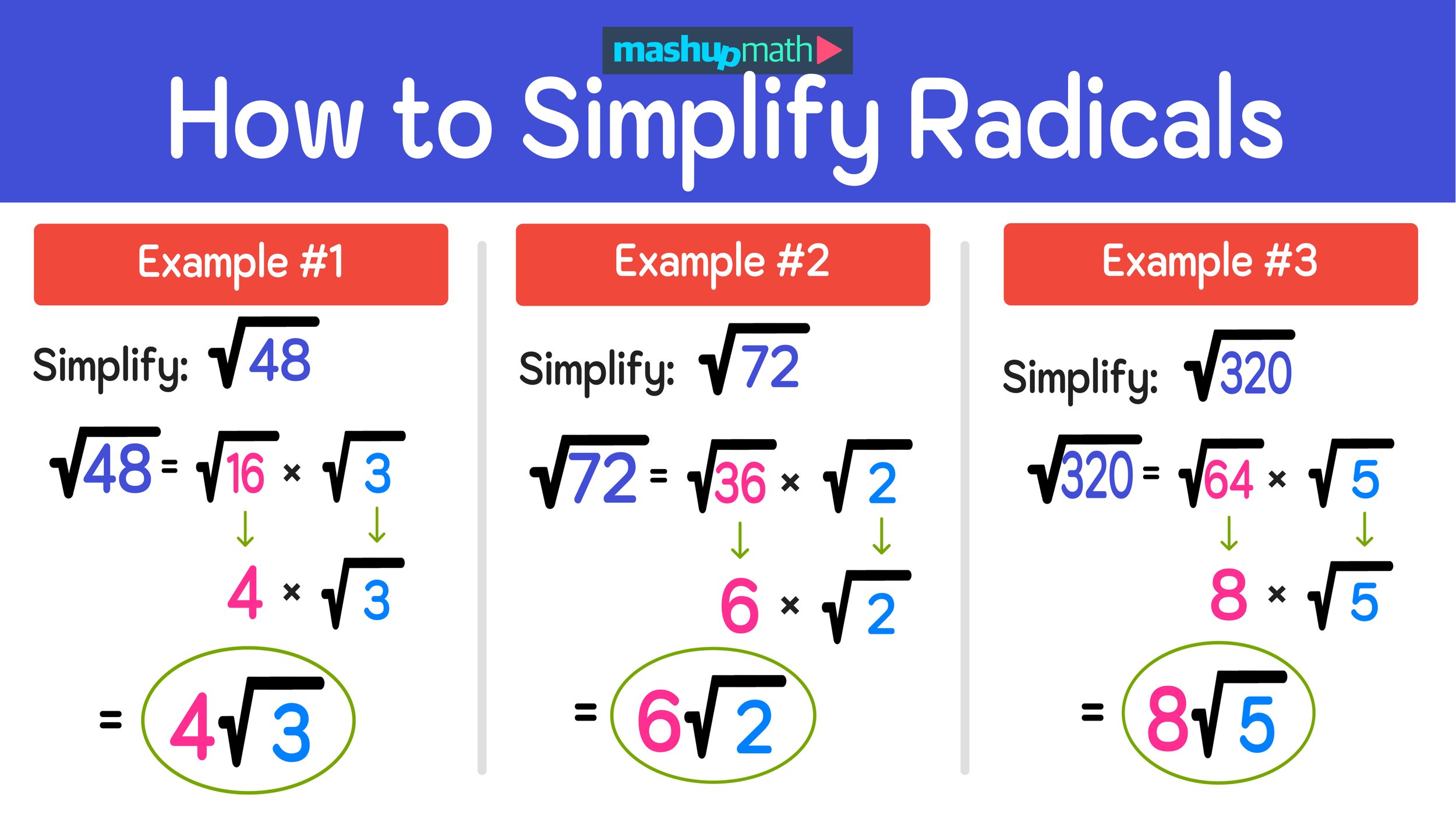
Example #2: Simplify √48
Just as we did in the previous example, we can use our 3-step strategy to simplify √48 as follows:
Step One: List all of the factors of the number inside of the radical
Let’s start by listing all of the factors of 48:
Factors of 48: 1, 2, 3, 4, 6, 8, 12, 16, 24, 48
Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.
For the next step, notice that 48 has two perfect square factors other than one: 4 and 16. Remember that, if we want to simplify completely, we must choose the largest perfect square factor, which, in this case, is 16.
16 is a factor of 48 and 16 is a perfect square
16 x 3 = 48
Step Three: Split the original radical into two radicals and simplify.
Lastly, we have to split the radical into the radicals of two of its factors where one of them is a perfect square (in this example, the perfect square factor is 16 and the non-perfect square factor is 3).
√48 = √(16 x 3) = √16 x √3
Since 16 is a perfect square (16 = 4x4), we can rewrite √16 as 4:
√48 = √16 x √3 = 4 x √3
And now we have our final answer:
Final Answer: √48 = 4√3
Figure 06 below shows how we used our 3-step strategy to simplify the radical √48.
Figure 06: How to Simply a Radical: √48 = 4√3
Are you feeling better about using our 3-step strategy to simplify radicals? Let’s move on and work through one more example.
How to Simplify Radicals Example #3
Example #3: Simplify √320
For this final example, we have to simplify a radical with a triple-digit non-perfect square inside of it: √320. We can do just that by using our 3-step strategy:
Step One: List all of the factors of the number inside of the radical
Start by listing all of the factors of the number inside of the radical, which, in this case, is 320:
Factors of 48: 1, 2, 4, 5, 8, 10, 16, 20, 32, 40, 64, 80, 160, 320
Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.
Next, identify all of the perfect square factors of 320 other than 1. In this case, we have 4, 16, and 64. The largest of these three perfect square factors is 64.
64 is a factor of 320 and 64 is a perfect square
64 x 5 = 320
Step Three: Split the original radical into two radicals and simplify.
For the very last step, we must split the radical √320 into the radicals of two of its factors. For this third example, the perfect square factor is 64 and the non-perfect square factor is 5.
√320 = √(64 x 5) = √64 x √5
Since 64 is a perfect square (64 = 8x9), we can rewrite √64 as 8:
√320 = √64 x √5 = 8 x √5
All that we left to do is conclude that:
Final Answer: √320 = 8√5
The graphic in Figure 07 below illustrates how we solved this problem using our 3-step strategy.
Figure 07: How to Simply Radicals: √320 = 8√5
Conclusion: How to Simplify Radicals in 3 Easy Steps
Whenever you have to simplify a radical with a non-perfect square inside, you have to find two factors of that number, one of which must be a perfect square. Once you find two factors that meet these conditions, you can simplify the perfect square and rewrite the radical in simplified form.
The process of simplifying radicals of non-perfect squares can done by following these three steps:
Step One: List all of the factors of the number inside of the radical
Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.
Step Three: Separate the original radical into two radicals and simplify.
As long as you can follow these steps, you can learn how to simplify radicals and you can solve a variety of problems.